View sample mathematical learning research paper. Browse research paper examples for more inspiration. If you need a psychology research paper written according to all the academic standards, you can always turn to our experienced writers for help. This is how your paper can get an A! Feel free to contact our writing service for professional assistance. We offer high-quality assignments for reasonable rates.
Does beauty have structure? How does a hinge work? What happens if zero divides a number? Do the symmetries of a triangle and the set of integers under addition have any structure in common? How many distinct patterns of wallpaper design are possible? What are Nature’s numbers? How do nurses determine the dosage of drugs (e.g., Pozzi, Noss, & Hoyles, 1998) or entomologists quantify relations among termites (e.g., Hall, Stevens, & Torralba, in press)? What forms of mathematical activity are found in automotive production (Smith, 1999)? Questions like these suggest the enormous imaginative scope and practical reach of mathematics and demonstrate that mathematicians are jugglers not of numbers, but of concepts (e.g., Stewart, 1975). Mathematical practice spans a universe of human endeavor, ranging from art and craft to engineering design, and its products extend over much of recorded history. Despite this long history of mathematics, systematic study of mathematical learning occupies only a brief slice in time. Nevertheless, research in mathematics education and in the psychology of mathematical learning continues to grow, so that any review of this research is necessarily incomplete and highly selective.
Academic Writing, Editing, Proofreading, And Problem Solving Services
Get 10% OFF with 24START discount code
Our choices for this review stem from a genetic view of knowledge (Piaget, 1970), a “commitment that the structures, forms, and possibly the content of knowledge is determined in major respects by its developmental history” (diSessa, 1995, p. 23). Mathematics develops within a collective history of argument and inscription (Davis & Hersh, 1981; Devlin, 2000; Kline, 1980; Lakatos, 1976; Nunes, 1999; Polya, 1945), so a genetic account of mathematical learning describes potential origins and developmental landscapes of these modes of thought. Accordingly, we first examine the nature of mathematical argument, tracing a path between everyday forms of argument and those that are widely recognized as distinctly mathematical. In this first section we focus on the epistemology (the grounds for knowing) and skills of argument, rather than on the more familiar heuristics and processes of mathematical reasoning (see, e.g., Haverty, Koedinger, Klahr, & Alibali, 2000; Leinhardt & Schwarz, 1997; Schoenfeld, 1992). We suggest that developmental roots of mathematical argument reside in the structure of narrative and pretend play but note how these roots must be nurtured to promote epistemic appreciation of proof and related forms of mathematical argument.
We next turn to the role that inscriptions (e.g., markings in a medium such as paper) and notations play in the growth and development of mathematical ideas. Our intention once again is to illuminate the developmental relationship between informal scratches on paper and the kinds of symbol systems employed in mathematical practice. In concert with the core role assigned to argument, we suggest that mathematical thinking emerges as refinement of everyday claims about pattern and possibility yet departs from these everyday roots as these claims are progressively inscribed and otherwise symbolized. Inscription and mathematical thinking co-originate (Rotman, 1993), so that mathematics emerges as a distinct form of literacy, much in the manner in which writing distinguishes itself from speech.
From these starting points we examine how these general qualities of mathematical thinking play out in two realms: geometry measurement and mathematical modeling. We chose the former because spatial mathematics typically receives short shrift in reviews of this kind, yet it encompasses a tradition that spans two millennia. Furthermore, spatial visualization is increasingly relevant to scientific inquiry and is undergoing a renaissance in contemporary computational mathematics. Modeling was selected as the second strand because modeling emphasizes the need for a broad mathematical education that includes several forms of mathematical inquiry. Moreover, modeling underscores the need to develop accounts of mathematical learning at the boundaries of professional practices and conventionally recognized mathematical activity (e.g., Moschkovich, 2002).
The studies selected for this review reflect both cognitive (e.g., Anderson & Schunn, 2000) and sociocultural perspectives (e.g., Forman, in press; Greeno, 1998) on learning. Studies of cognitive development typically shed light on individual cognitive processes, for example, how young students might think about units of measure and how their understandings might evolve. In contrast, sociocultural perspectives typically underscore thinking as mediated activity (e.g., Mead, 1910; Wertsch, 1998). For example, one might consider the history of cultural artifacts, such as rulers, in children’s developing conceptions of units. We believe that both forms of analysis are indispensable and that, in fact, these perspectives are interwoven for learners, regardless of researchers’proclivities to consider them as distinct enterprises. Consider, for example, the idea of learning to construct a geometric proof. On the one hand, a cognitive analysis characterizes the kinds of skills required to develop a proof and describes how those skills must be orchestrated (e.g., Koedinger & Anderson, 1990). These forms of characterization seem indispensable to instructional design (Anderson & Schunn, 2000). On the other hand, the need for proof is cultural, arising from an epistemology that values proof as explanation (Harel & Sowder, 1998; Hersh, 1993). Accordingly, this perspective poses the challenge not just of accounting for the understanding of proof, but also of how one might inculcate a classroom culture that values proof. In the sections that follow, we attempt to strike a balance between these two levels of explanation because both supply important accounts of mathematical learning. Because we assume that readers are familiar with the general nature of these two kinds of analysis, we will not flesh out the assumptions of each perspective in this research paper.
The Growth Of Argument
Arguments in mathematics aim to provide explanations of mathematical structures. Proof is often taken as emblematic of mathematical argument because it both explains and provides grounds for certainty that are hard to match or even imagine in other disciplines, such as science or history. Although everyday folk psychology often associates proof with drudgery, for mathematicians proof is a form of discovery (e.g., de Villiers, 1998), and even “epiphany” (e.g., Benson, 1999). Yet conviction does not start with proof, so in this section we trace the ontogeny of forms of reasoning that seem to ground proof and proof-like forms of explanation. Our approach here is necessarily speculative because there is no compelling study of the long-term development of an epistemic appreciation for mathematical argument. Moreover, the emblem of mathematical argument, proof, is often misunderstood as a series of conventional procedures for arriving at the empirically obvious, rather than as a form of explanation (Schoenfeld, 1988). International comparisons of students (e.g., Healy & Hoyles, 2000) confirm this impression, and apparently many teachers hold similar views (Knuth, 2002; Martin & Harel, 1989).
Nonetheless, several lines of research suggest fruitful avenues for generating an epistemology of mathematical argument that is more aligned with mathematical practice and more likely to expose progenitors from which this epistemology can be developed. (We are not discounting the growth of experimental knowledge in mathematics but are focusing on grounds for certainty here. We return to this point later.) In the sections that follow, we suggest that mathematical argument evolves from everyday argument and represents an epistemic refinement of everyday reasoning. We propose that the evolution is grounded in the structure of everyday conversation, is sustained by the growth and development of an appreciation of pretense and possibility, and is honed through participation in communities of mathematical inquiry that promote generalization and certainty.
Conversational Structure as a Resource for Argument
Contested claims are commonplace, of course, and perhaps there is no more common arena for resolving differing perspectives than conversation. Although we may well more readily recall debates and other specialized formats as sparring grounds, everyday conversation also provides many opportunities for developing “substantial” arguments (Toulmin, 1958). By substantial, Toulmin referred to arguments that expand and modify claims and propositions but that lead to conclusions not contained in the premises (unlike those of formal logic). For example, Ochs, Taylor, Rudolph, and Smith (1992) examined family conversations with young children (e.g., 4–6 years of age) around such mundane events as recall of “the time when” (e.g., mistaking chili peppers for pickles) or a daily episode, such as an employee’s reaction to time off. They suggested that dinnertime narratives engender many of the elements of sound argument in a manner that parallels scientific debate. First, narratives implicate a problematic event, a tension in need of resolution, so that narratives often embody some form of contest, or at least contrast. Second, the problematic event often invites causal explanation during the course of the conversation. Moreover, these explanations may be challenged by conarrators or listeners, thus establishing a tacit anticipation of the need to ground claims. Challenges in everyday conversations can range from matters of fact (e.g., disputing what a character said) to matters of ideology (e.g., disputing the intentions of one of the characters in the account). Finally, conarrators often respond to challenges by redrafting narratives to provide alternative explanations or to align outcomes more in keeping with a family’s worldview. By means of explicit parallels like these, Ochs et al. (1992) argued that theories and stories may be generated, critiqued, and revised in ways that are essentially similar (see Hall, 1999; Warren, Ballanger, Ogonowski, Rosebery, & Hudicourt-Barnes, 2001, regarding continuities between everyday and scientific discourses).
Studies like those of Ochs et al. (1992) are emblematic of much of the work in conversation analysis, which suggests that the structure of everyday talk in many settings is an important resource for creating meaning (Drew & Heritage, 1992). For example, Rips (1998; Rips, Brem, & Bailenson, 1999) noted that everyday conversationalists typically make claims, ask for justification of others’ claims, attack claims, and attack the justifications offered in defense of a claim. The arrangement of these moves gives argumentation its characteristic shape. Judgments of the informal arguments so crafted depend not only on the logical structure of the argument but also on consideration of possible alternative states of the claims and warrants suggested. Rips and Marcus (1976) suggested that reasoning about such suppositions, or possible states, requires bracketing uncertain states in memory in order to segregate hypothetical states from what is currently believed to be true. In the next section we review evidence about the origins and constraints on this cognitive capacity to reason about the hypothetical.
From Pretense to Proof
Reasoning about hypothetical states implicates the development of a number of related skills that culminate in the capacity to reason about relations between possible states of the world, to treat aspects of them as if they were in the world, to objectify possibilities, and to coordinate these objects (e.g., conjectures, theories, etc.) with evidence. Both theory and evidence are socially sanctioned and thus cannot be properly regarded apart from participation in communities that encourage, support, and otherwise value these forms of reasoning. We focus first on the development of representational competence, which appears to originate in pretend play, and then on corresponding competencies in conditional reasoning. We turn then from competence to dispositions to construct sound arguments that coordinate theory and evidence and, in mathematics, to prove. Because these dispositions do not seem to arise as readily as the competencies that underlie them, we conclude with an examination of the characteristics of classroom practices that seem to support the development of generalization and grounds for certainty in early mathematics education.
Development of Representational Competence
One of the features of mathematical argument is that one must often reason about possible states of affairs, sometimes even in light of counterfactual evidence. As we have seen, this capacity is supported by everyday conversational structure. However, such reasoning about possibility begins with representation. This representational capacity generally emerges towards the end of the second year and is evident in children’s pretend play. Leslie (1987) clarified the representational demands of pretending that a banana is a telephone, while knowing very well that whatever the transformation, the banana remains a banana, after all. He suggested that pretense is founded in metarepresentational capacity to constitute (and distinguish) a secondary representation of one’s primary representation of objects and events.
Metarepresentation expands dramatically during the preschool years. Consider, for example, DeLoache’s (1987, 1989, 1995) work on children’s understanding of scale models of space. DeLoache encouraged preschoolers to observe while she hid small objects in a scale model of a living room. Then she brought them into the full-scale room and asked them to find similar objects in the analogous locations. DeLoache observed a dramatic increase in representational mapping between the model and the world between 2.5 and 3 years of age. Younger children did not seem to appreciate, for example, that an object hidden under the couch in the model could be used to find its correspondent in the room, even though they readily described these correspondences verbally. Yet slightly older children could readily employ the model as a representation, rather than as a world unto itself, suggesting that they could sustain a clear distinction between representation and world.
Gentner’s (Gentner & Loewenstein, 2002; Gentner & Toupin, 1986) work on analogy also focuses on early developing capacities to represent relational structures, so that one set of relations can stand in for another. For example, Kotovsky and Gentner (1996) presented triads of patterns to children ranging from 4 to 8 years of age. One of the patterns was relationally similar to an initially presented pattern (e.g., small circle, large circle, small circle matched to small square, large square, small square), and the third was not (e.g., large square, small square, small square). Although the 4-year-olds responded at chance levels, 6- and 8-year-olds preferred relational matches. These findings are consistent with a relational shift from early reliance on object-matching similarity to later capacity and preference for reasoning relationally (Gentner, 1983). This kind of relational capacity undergirds conceptual metaphors important to mathematics, like those between collections of objects and sets in arithmetic, and forms the basis for the construction of mathematical objects (Lakoff & Nunez, 2000). Moreover, Sfard (2000) pointed out that although discourse about everyday events and objects is a kind of first language game (in Wittgenstein’s sense), the playing field in mathematics is virtual, so that mathematical discourse is often about objects that have no counterpart in the world.
Knitting Possibilities: Counterfactual Reasoning
Collectively, research on the emergence of representational competence illuminates the impressive cognitive achievement of creating and deploying representational structures of actual, potential, and pretend states of the world. However, it is yet another cognitive milestone to act on these representations to knit relations among them, a capacity that relies on reasoning about relations among these hypothetical states. Children’s ability to engage in such hypothetical reasoning is often discounted, perhaps because the seminal work of Inhelder and Piaget (1958) stressed children’s, and evenadults’, difficulties with the (mental) structures of logical entailment. However, these difficulties do not rule out the possibility that children may engage in forms of mental logic that provide resources for dealing with possible worlds, even though they may fall short of an appreciation of the interconnectedness of mental operators dictated by formal logic. Studies of child logic document impressive accomplishments even among young children. For the current purpose of considering routes to mathematical argument, we focus on findings related to counterfactual reasoning—reasoning about possible states that run counter to knowledge or perception, yet are considered for the sake of the argument (Levi, 1996; Roese, 1997). This capacity is at the heart of deductive modes of thought that do not rely exclusively on empirical knowledge, yet can be traced to children’s capacity to coordinate separate representations of true and false states of affairs in pretend play (Amsel & Smalley, 2001).
In one of the early studies of young children’s hypothetical reasoning, Hawkins, Pea, Glick, and Scribner (1984) asked preschool children (4 and 5 years) to respond to syllogistic problems with three different types of initial premises: (a) congruent with children’s empirical experience (e.g., “Bears have big teeth”), (b) incongruent with children’s empirical experience (e.g., “Everything that can fly has wheels”), and (c) a fantasy statement outside of their experience (e.g., “Every banga is purple”). Children responded to questions posed in the syllogistic form of modus ponens (“Pogs wear blue boots. Tom is a pog. Does Tom wear blue boots?”). They usually answered the congruent problems correctly and the incongruent problems incorrectly. Furthermore, children’s responses to incongruent problems were consistent with their experience, rather than the premises of the problem. This empirical bias was a consistent and strong trend. However, unexpectedly, when the fantasy expressions were presented first, children reasoned from premises, even if these premises contradicted their experiences. This finding suggested that the fantasy form supported children in orienting to the logical structure of the argument, rather than being distracted by its content.
Subsequently, Dias and Harris (1988, 1990) presented young children (4-, 5- and 6-year olds) with syllogisms, some counterfactual, such as, “All cats bark. Rex is a cat. Does Rex bark?” When they were cued to treat statements as make-believe, or when they were encouraged to imagine the states depicted in the premises, children at all ages tended to reason from the premises as stated, rather than from their knowledge of the world. Scott, Baron-Cohen, and Leslie (1999) found similar advantages of pretense and imagination with another group of 5-year-old children as well as with older children who had learning disabilities. Harris and Leevers (2001) suggested that extraordinary conditions of pretense need not be invoked. They obtained clear evidence of counterfactual reasoning with preschool children who were simply prompted to think about the content of counterfactual premises or, as they put it, to adopt an analytic perspective.
Further research of children’s understandings of the entailments of conditional clauses suggests that at or around age 8, many children interpret these clauses biconditionally. That is, they treat the relationship symmetrically (Kuhn, 1977; Taplin, Staudenmayer, & Taddonio, 1974), rather than treating the first clause as a sufficient but not necessary condition for the consequent (e.g., treating “if anthrax, then bacteria” as symmetric). However, Jorgenson and Falmagne (1992) assessed 6-year-old children’s understanding of entailment in story formats and found that this form of narrative support produced comprehension of entailment more like that typically shown by adults. O’Brien, Dias, Roazzi, and Braine (1998) suggested that the conflicting conclusions like these about conditional reasoning can be traced to the model of material implication (if P, then Q) based on formal logic. O’Brien and colleagues argued that it may be a mistake to evaluate conditional reasoning via the truth table of formal logic (especially the requirement that a conditional is true whenever its antecedent is false). This perspective, they think, obscures the role of conditionals in ordinary reasoning. They proposed instead that a set of logic inference schemas governs conditional reasoning. Collectively, these schemas rely on supposing that the antecedent is true and then generating the truth of the consequent. They found that second- and fifth-grade children in both the United States and Brazil could judge the entailments of the premises of a variety of conditionals (e.g., P or Q, Not-P or Not-Q) in ways consistent with these schemas, rather than strict material implication. Even preschool children judged a series of counterfactual events, for example, those that would follow from a character pretending to be a dog, as consistent with a story.An interesting result was that they also excluded events that were suppositionally inconsistent with the story, for example, the same character talking on the phone even though those events were presumably more consistent with their experience (i.e., people, not dogs, use phones).
Collectively, these studies of hypothetical reasoning point to an early developing competence for representing and comparing possible and actual states of the world, as well as for comparing possible states with other possible states. Moreover, these comparisons can be reasoned about in ways that generate sound deductions that share much, but do not overlap completely, with formal logic. These impressive competencies apparently arise from the early development of representational competence, especially in pretend play (Amsel & Smalley, 2001), as well as the structure of everyday conversation. However, despite these displays of early competence, other work suggests that the skills of argument are not well honed at any age, and are especially underdeveloped in early childhood.
The Skills of Argument
Kuhn (1991) suggested that an argument demands not only generation of possibilities but also comparison and evaluation of them. These skills of argument demand a clear separation between beliefs and evidence, as well as development of the means for establishing systematic relations between them (Kuhn, 1989). Kuhn (2001) viewed this development as one of disposition to use competencies like those noted, a development related to people’s epistemologies: “what they take it to mean to know something” (Kuhn, 2001, p. 1). In studies with adults and adolescents (ninth graders) who attempted to develop sound arguments for the causes of unemployment, school failure, and criminal recidivism, most of those interviewed did not seem aware of the inherent uncertainty of their arguments in these ill-structured domains (Kuhn, 1991, 1992). Only 16% of participants generated evidence that would shed light on their theories, and only about one third were consistently able to generate counterarguments to their positions. Kuhn, Amsel, and O’Loughlin (1988) found similar trends with people ranging in age from childhood (age 8) to adulthood who also attempted to generate theories about everyday topics like the role of diet in catching colds. Participants again had difficulty generating and evaluating evidence and considering counterarguments.
Apparently, these difficulties are not confined to comparatively ill-structured problems. For example, in a study of the generality and specificity of expertise in scientific reasoning, Schunn and Anderson (1999) found that nearly a third of college undergraduate participants never supported their conjectures about a scientific theory with any mention of empirical evidence. Kuhn (2001) further suggested that arguments constructed in contexts ranging from science to social justice tend to overemphasize explanation and cause at the expense of evidence and, more important, that it is difficult for people at all ages to understand the complementary epistemic virtues of each (understanding vs. truth).
Proof
The difficulties that most people have in developing epistemic appreciations of fundamental components of formal or scientific argument suggest that comprehension and production of more specialized epistemic forms of argument, such as proof, might be somewhat difficult to learn. A number of studies confirm this anticipation. For example, Edwards (1999) invited 10 first-year high school students to generate convincing arguments about the truth of simple statements in arithmetic, such as, “Even x odd makes even.” The modal justification was, “I tried it and it works” (Edwards, p. 494). When pressed for further justification, students resorted to additional examples. In a study of 60 high school students who were invited to generate and test conjectures about kites, Koedinger (1998) noted that “almost all students seemed satisfied to stop after making one or a few conjectures from the example(s) they had drawn” (p. 327). Findings like these have prompted suggestions that “it is safer to assume little in the way of proof understanding of entering college students” (Sowder, 1994, p. 5).
What makes proof hard? One source of difficulty seems to be instruction that emphasizes formalisms, such as twocolumn proofs, at the expense of explanation (Coe & Ruthven, 1994; Schoenfeld, 1988). Herbst (2002) went so far as to suggest that classroom practices like two-column proofs often bind students and their instructors in a pedagogical paradox because the inscription into columns embodies two contradictory demands. The format scripts students’ responses so that a valid proof is generated. Yet this very emphasis on form obscures the rationale for the choice of the proposition to be proved: Why is it important to prove the proposition so carefully? What does the proof explain? Hoyles (1997; Healy & Hoyles, 2000) added that curricula are often organized in ways that de-emphasize deductive reasoning and scatter the elements of proof across the school year (see also Schoenfeld, 1988, 1994).
In their analysis of university students’ conceptions of proof, Harel and Sowder (1998) found that many students seem to embrace ritual and symbolic forms that share surface characteristics with the symbolism of deductive logic. For example, many students, even those entering the university, appear to confuse demonstration and proof and therefore value a single case as definitive. Martin and Harel (1989) examined the judgments of a sample of preservice elementary teachers enrolled in a second-year university mathematics course. Over half judged a single example as providing a valid proof. Many did not accept a single countercase as invalidating a generalization, perhaps because they thought of mathematical generalization as a variation of the generalizations typical of prototypes of classes (e.g., Rosch, 1973). Outcomes like these are not confined to prospective teachers: Segal (2000) noted that 40% of entry-level university mathematics students also judged examples as valid proofs.
Although many studies emphasize the logic of proof, others examine proof as a social practice, one in which acceptability of proof is grounded in the norms of a community (e.g., Hanna, 1991, 1995). These social aspects of proof suggest a form of rationality governed by artifacts and conventions about evidence, rigor, and plausibility that interact with logic (Lakatos, 1976; Thurston, 1995). Segal (2000) pointed out that conviction (one’s personal belief) and validity (the acceptance of this belief by others) may not always be consistent. She found that for first-year mathematics students, these aspects of proof were often decoupled. This finding accords well with Hanna’s (1990) distinction between proofs that prove and those that explain, a distinction reminiscent of Kuhn’s (2001) contrast between explanation and evidence. Chazan (1993) explored the proof conceptions of 17 high school students from geometry classes that emphasized empirical investigation as well as deductive proof. Students had many opportunities during instruction to compare deduction and induction over examples. One component of instruction emphasized that measurement of examples may suffer from accuracy and precision limitations of measurement devices (such as the sum of the angles of triangles drawn on paper). A second component of instruction highlighted the risks of specific examples because one does not know if one’s example is special or general. Nevertheless, students did not readily appreciate the virtues of proof. One objection was that examples constituted a kind of proof by evidence, if one was careful to generate a wide range of them. Other students believed that deductive proofs did not provide safety from counterexamples, perhaps because proof was usually constructed within a particular diagram.
Harel (1998) suggested that many of these difficulties can be traced to fundamental epistemic distinctions that arose during the history of mathematics. In his view, students’ understanding of proof is often akin to that of the Greeks, who regarded axioms as corresponding to ideal states of the world (see also Kline, 1980). Hence, mathematical objects determine axioms, but in a more modern view, objects are determined by axioms. Moreover, in modern mathematics, axioms yield a structure that may be realized in different forms. Hence, students’efforts to prove are governed by epistemologies that have little in common with those of the mathematicians teaching them, a difficulty that is both cultural and cognitive. Of course, the cultural-epistemic obstacles to proof are not intended to downplay cognitive skills that students might need to generate sound proofs (e.g., Koedinger, 1998). Nevertheless, it is difficult to conceive of why students might acquire the skills of proof if they do not see its epistemic point.
Reprise of Pretense to Proof
The literature paints a somewhat paradoxical portrait of the development of mathematical argument, especially the epistemology of proof. On the one hand, mathematical argument utilizes everyday competencies, like those involved in resolving contested claims in conversation and those underlying the generation and management of relations among possible states of the world. On the other hand, mathematical argument invokes a disposition to separate conjectures from evidence and to establish rigorous relations between them— all propensities that appear problematic for people at any age. Moreover, the emphasis on structure and certainty in mathematics appears to demand an epistemological shift away from things in the world to structures governed by axioms that may not correspond directly to any personal experience, except perhaps by metaphoric extension (e.g., Lakoff & Nunes, 1997). To these cognitive burdens we can also safely assume that the practices from which this specialized form of argument springs are hidden, both from students and even (within subfields of mathematics) from mathematicians themselves (e.g., Thurstone, 1995). Despite this paradox, or perhaps because of it, emerging research suggests a synthesis where the everyday and the mathematical can meet, so that mathematical argument can be supported by—yet differentiated from—everyday reasoning. In the next section we explore these possibilities.
Mathematical Argument Emerges in Classrooms That Support It
As the previous summary illustrates, research generally paints a dim portrait of dispositions to create sound arguments, even in realms less specialized than mathematics. Nonetheless an emerging body of research suggests a conversational pathway toward developing mathematical argument in classrooms. The premise is that classroom discourse can be formatted and orchestrated in ways that make the grounds of mathematical argument visible and explicit even to young children, partly because everyday discourse offers a structure for negotiating and making explicit contested claims and potential resolutions (e.g., Wells, 1999), and partly because classrooms can be designed so that “norms” (e.g., Barker & Wright, 1954) of participant interaction can include mathematically fruitful ideas such as the value of generalization. Rather than treating acceptance or disagreement solely as internal states of mind, these are externalized as discursive activities (van Eemeren et al., 1996). A related claim is that classrooms can be designed as venues for initiating students in the “register” (Halliday, 1978; Pimm, 1987) or “Discourse” (Gee, 1997, in press) of a discipline like mathematics.
Dialogue, then, is a potential foundation for supporting argument, and studies outside of mathematics suggest that sound arguments can be developed in dialogic interaction. For example, Kuhn, Shaw, and Felton (1997) asked adolescents and young adults to create arguments for or against capital punishment. Compared to a control condition limited to repeated (twice) elicitation of their views, a group engaged in dyadic interactions (one session per week for five weeks) was much more likely to create arguments that addressed the desirability of capital punishment within a framework of alternatives. Students in this dyadic group also were more likely to develop a personal stance about their arguments. The development of argument in the engaged group was not primarily related to hearing about the positions of others, but rather to the need to articulate one’s own position, which apparently instigated voicing of new forms of argument. Moreover, criteria by which one might judge the desirability of capital punishment were elaborated and made more explicit by those participating in the dyadic conversations.
Studies of argument in classrooms where it is explicitly promoted are also encouraging. For example, Anderson, Chinn, Chang, Waggoner, and Yi (1997) examined the logical integrity of the arguments developed by fourth-grade children who participated in discussions about dilemmas faced by characters in a story. The discussions were regulated by norms of turn taking (students spoke one at a time and avoided interrupting each other), attentive listening, and the expectation of respectful challenge. The teacher’s role was to facilitate student interaction but not to evaluate contributions. Anderson et al. (1997) analyzed the microstructure of the resulting classroom talk. They found that children’s arguments generally conformed to modus ponens (if p, then q) if unstated but shared premises of children were taken into account. This context of shared understandings, generated from collective experiences and everyday knowledge, resolved referential ambiguities and thus constituted a kind of sound, conversational logic. However, “only a handful of children were consistently sensitive to the possibility of backing arguments with appeals to general principles” (Anderson et al., 1997, p. 162). Yet, such an emphasis on the general is an important epistemic component of argument in mathematics, which suggests that mathematics classrooms may need to be more than incubators of dialogue and the general norms that support conversational exchange.
Mathematical Norms
Cobb and his colleagues have conducted a series of teaching experiments in elementary school classrooms that examine the role of conversational norms more explicitly attuned to mathematical justification, such as those governing what counts as an acceptable mathematical explanation (e.g., Cobb, Wood, Yackel, & McNeal, 1992; Cobb, Yackel, & Wood, 1988; Yackel & Cobb, 1996). Cobb and his colleagues suggested that mathematical norms constitute an encapsulation of what counts as evidence, and a proliferation of norms suggests that students in a class are undertaking a progressive refinement and elaboration of mathematical meaning.
In this research several conversational gambits appear reliably to frame the emergence of mathematically fruitful norms. One is discussion of what constitutes a mathematical difference, prompted by teachers who ask if anyone has solved a problem in a different way. Yackel and Cobb (1996) described interactions among students and teachers solving number sentences like 78 ‒ 53 = ____. During the course of this interaction the teacher accepted strategies that involved recomposition or decomposition of numbers as different, but simple restatements of a particular strategy were not accepted as different (e.g., similar counts with fingers vs. teddy bears). The need to contribute to this kind of collective activity prompted students to reflect about how their strategy was similar to or different from those described by classmates, a step toward generalization. Moreover, McClain and Cobb (2001) found that negotiation of norms such as what counted as a mathematical difference among first-graders also spawned other norms such as what counted as a sophisticated solution. This cascade of norms appeared to have more general epistemological consequences, orienting children toward mathematics as pattern as they discovered relationships among numbers.
Hershkowitz and Schwarz (1999) tracked the arguments made by sixth-grade students in small group and collective discussions of solution strategies and also noted steps toward mathematics as pattern via discussion of mathematical difference. They observed that pedagogy in the sixth-grade class they studied was oriented toward “purifying” students’ invented strategies by suppressing surface-level differences among those proposed. The resulting distillation focused student attention on meaningful differences in mathematical structures. Here again a negotiation of what counted as a mathematical difference inspired the growth of mathematical thinking.
Krummheuer (1998) suggested that mathematical norms such as difference operate by formatting mathematical conversation, meaning that they frame the interactions among participants. Krummheuer (1995) proposed that formatting is consequential for learning because similarly formatted arguments invite cognitive recognition of similarity between approaches taken in these arguments, thus setting the stage for the distillation or purification noted previously. For example, Krummheuer (1995) documented how two second-grade boys initially disagreed about the similarity of their solution methods to the problem of 8 4, but later found that although one subtracted four from a previous result (9 4) and another eight from a previous result (10 4), they were really talking about the “same way.” This realization initiated discovery of what made them the same—a quality that, in turn, was staged by the norm of what counted as different.
Teacher Orchestration of Mathematical Conversation
The work of the teacher to establish norms is by no means clear-cut because privileging certain forms of explanation may compete with other goals, such as including all students. Hence, part of the work of the teacher is to find ways to orchestrate discussions that make norms explicit while also developing means to make a norm work collectively (McClain& Cobb, 2001). In her study of argumentation in a second-grade classroom, Wood (1999) illustrated the important role played by teachers in formatting participation itself. She traced how a second-grade teacher apprenticed students to the discourse of mathematical disagreement, differentiating this kind of disagreement from everyday, personal contest. Children apprenticed in problem-solving contexts well within their grasp, so that when they later disagreed about the meaning of place value (one student counted by tens from 49 and another disagreed, contending that counts had to start at decades, as in 50, 60, etc.), the resulting argument centered around mathematical,notpersonal,claims.Woodcautionedthatwhatmight seem like fairly effortless ability to orchestrate arguments about mathematical difference relies instead on prior spadework by the teacher. In this instance, much of that spadework revolved around formatting disagreement. Other classroom studies indicate that teachers assist mathematical argument by explicit support of suppositional reasoning. For example, Lehrer, Jacobson, et al. (1998) conducted a longitudinal study of second-grade mathematics teachers who increasingly encouraged students to investigate the implications of counterfactual propositions (e.g., “What would happen if it were true?”).
The work of the teacher to develop norms and format argument is part of a more general endeavor to understand how teachers assist student thinking about mathematics dialogically. Henningsen and Stein (1997) found that student engagement in classroom mathematics was associated with a sustained press for justification, explanations, or meaning through teacher questioning, comments, and feedback. Spillane and Zeuli (1999) noted that despite endorsing mathematics reform, teachers nevertheless had difficulty orienting conversation in the classroom toward significant mathematical principles and concepts.
O’Connor and Michaels (1996) suggested that teacher orchestration of classroom conversations “provides a site for aligning students with each other and with the content of the academic work while simultaneously socializing them into particular ways of speaking and thinking” (p.65). The conversational mechanisms by which teachers orchestrate mathematically productive arguments include “revoicing” student utterances so that teachers repeat, expand, rephrase, or animate these parts of conversation in ways that increase their scope or precision or that juxtapose temporally discrete claims for consideration (O’Connor & Michaels, 1993, 1996). For example, a student may explain how she solved a perimeter problem by saying that she counted all around the hexagonal shape. In response, her teacher might rephrase the student’s utterance by substituting “perimeter” for her expression “all around.” In this instance, the teacher is substituting a mathematical term, “perimeter,” for a more familiar, but imprecise construction, “all around,” thereby transforming the student’s utterance spoken in everyday language into mathematical reference (Forman, Larreamendy-Joerns, Stein, & Brown, 1998).
Revoicing encompasses more complex goals than substitution of mathematical vocabulary for everyday words or even expanding the range of a mathematical concept. Some revoicing appears to be aimed at communicating respect for ideas and at the larger epistemic agenda of helping students identify aspects of mathematical activity, such as the need to “know for sure” or the idea that a case might be a window to a more general pattern (Strom, Kemeny, Lehrer, & Forman, 2001). For example, in a study of second graders who were learning about geometric transformations by designing quilts, Jacobson and Lehrer (2000) examined differences in how teachers revoiced children’s comments about an instructional video that depicted various kinds of geometric transformations in the context of designing a quilt. They found an association between teacher revoicing and student achievement. In classes where teachers revoiced student comments in ways that invited conjectures about the causes of observed patterns or that drew attention to central concepts, students’ knowledge of transformational geometry exceeded that of counterparts whose teachers merely paraphrased or repeated student utterances.
Pathways to Proof
In classroom cultures characterized by cycles of conjecture and revision in light of evidence, student reasoning can become quite sophisticated and can form an important underlying foundation for the development of proof (Reid, 2002). For example, Lampert (2001; Lampert, Rittenhouse, & Crumbaugh, 1996) described a classroom argument about a claim made by one student that 13.3 was one fourth of 55. Other students claimed, and the class accepted, 27.5 as one half of 55. Another student noted that 13.3 13. 3 26.6, with the tacit premise that one fourth and one fourth is one half, and hence refuted the first claim. Lampert (2001) noted that the logical form of this proof also served to generate an orientation toward student authority and justification, so that the teacher (Lampert) was not the sole or even chief authority on mathematical truth. Ball and Bass (2000) documented a similar process with third-grade students who worked from contested claims to commonly accepted knowledge by processes of conjecturing, generating cases, and “confronting the very nature and challenge of mathematical proof” (p. 196).
Although generating conjectures and exploring their ramifications is an important precursor to proof, ironically it is grasping the limitations of this form of argument that motivates an important development toward proof as necessity. In classrooms like those taught by Lampert and by Ball, the need for proof emerges as an adjunct to sound argument. For example, a pair of third graders working on a conjecture that an odd number plus an odd number is an even number generated many cases consistent with the conjecture. Yet they were not satisfied because, as one of them said: “You can’t prove that Betsy’s conjecture always works. Because um, there’s, um like, numbers go on and on forever and that means odd numbers and even numbers go on forever, so you couldn’t prove that all of them aren’t” (Ball & Bass, 2000, p. 196).
Children’s recognition of the limits of case-based induction has also been observed in other classrooms where teachers orchestrate discussions and develop classroom cultures consistent with mathematical practices. For example, Lehrer, Jacobson, et al. (1998) observed a class of second-grade students exploring transformational geometry who developed the conjecture that there would always be some transformation or composition of transformations that could be applied to an asymmetric cell (a core unit) that would result in a symmetric design. The class searched vigorously for a single countercase among all the asymmetric core units designed by the children in this class and could not generate any refutation. Nevertheless, a subset of the class remained unconvinced and continued to insist that that they could not “be really sure.” Their rationale, like that of the third grader described earlier, focused on the need to exhaustively test all possible cases, a need that could not be met because “we’d have to test all the core squares in the world that are asymmetric” (Lehrer, Jacobson, et al., 1998, p. 183). They went on to note that this criterion could not possibly be met due to its infinite size and also because “people are probably making some right now” (p. 183). Hence, in classrooms like these, the need for proof arises as children recognize the limitations of the generalization of their argument. Of course, such need arises only when norms valuing generalization and its rationale are established.
When children have the opportunity to participate regularly in these kinds of classroom cultures, there is good evidence that their appreciation of mathematical generalization and the epistemology of proof take root (e.g., Kaput, 1999). For example, Maher and Martino (1996) traced the development of one child’s reasoning over a five-year span (Grade 1 through 5) as she participated in classrooms of literate mathematical practice. A trace of conceptual change was obtained by asking Stephanie to figure out how many different towers four or five cubes tall can be made if one selects among red and blue cubes. In the third grade Stephanie attempted to generate cases of combination and eliminate duplicates. Her justification for claiming that she had found all possible towers was that she could not generate any new ones. By the spring of the fourth grade, Stephanie was no longer content with mere generation and instead constituted an empirical proof by developing a means for exhaustively searching all possibilities.
In another longitudinal study (Grades 2–3), Lehrer and his colleagues followed students in the same second-grade class that had discovered the limits of case-based generalization into and over the course of the third grade. These students’ mathematical experiences continued in a classroom emphasizing conjecture, justification, and generalization. Over the course of the third-grade year, researchers recorded many instances of student-generated proof in the context of classroom discussion. At the end of the third grade, all children in the class were interviewed about their pBibliography: for justifications of mathematical conjectures to determine whether proof genres sustained in classroom dialogues would guide the thinking of individual students (Strom & Lehrer, 1999).
Four conjectures were presented in the interview, two of which were false and two of which were true. Justifications for true conjectures included single cases, multiple cases, simple restatement of the conjecture in symbolic notation, abstraction of single cases (notation without generalization, as in using an abstract pattern of dots to represent the commutative property of a case), and valid generalizations, in the form of visual proofs (e.g., the rotational invariance of an arbitrary rectangle for commutative property of multiplication). The range of justification types was designed to distinguish between case-based and deductive generalizations on the one hand and the form of proof (the restatement of the conjecture in symbolic notation) from its substance on the other. A similar format of justifications was employed for false conjectures, such as, “When you take half of an even number, you get an even number. ”Here, however, we also included a single counterexample. Students rank-ordered their preferences. For the false conjecture, over half (55%) of the students selected the counterexample as the best justification and the single case as the worst. For the true conjectures, the majority chose the visual proof as best and either the single case or simple translation of the statement into symbolic notation as worst.
Strom and Lehrer (1999) also observed processes of proof generation for these 21 students, asking students to prove that two times any number is an even number. Two of the 21 students rejected the claim immediately, citing counterexamples with fractions (we had intended whole numbers as a tacit premise). Three other students cited the problem of proof by induction, generated several cases, noted that they were “pretty sure” that the conjecture was true, and then decided that they could not prove it because, as one put it, “because the numbers never stop. . . . I couldn’t ever really prove that” (p. 31). Other students (n 3) followed a similar line of reasoning, suggesting that they had “proved it to myself, but not for others” (p. 32). Five students solved the problem of induction, either by drawing on definition to deduce the truth of the conjecture or by describing how the patterns they noticed from exploring several cases constituted a pattern that could be applied to all numbers. For example, two of these five students verified the conjecture for the numbers 1 through 10 and then stated that for numbers greater than 10 “any number that ends in an even number is even” (p. 32). Then each student showed how this implied that the pattern of even numbers they had verified for 1 through 10 extended to all numbers— “The rest of the numbers just have a different number at the beginning” (p. 32). The remaining students generated several cases, searched for and failed to find counterexamples, and then declared that they saw the pattern and so believed the conjecture true. In summary, students who had repeated opportunity to construct generalizations and proofs during the course of classroom instruction were sensitive to the role of counterexamples in refutation, and nearly all appreciated the limitations of relying on cases (unless one could exhaustively search the set). Generation of proof without dialogic assistance was considerably more difficult, but in fact, many were capable of constructing valid proofs, albeit with methods considerably more limited than those at the disposal of participants well versed in the discipline.
In well-constituted classrooms, young students can succeed at these forms of reasoning with appropriate assistance. However, work with adults illustrates how difficult it can be to acculturate students to proof-based argument. Simon and Blume (1996) conducted a study of prospective mathematics teachers who were schooled traditionally. At first, students were satisfied by induction over several cases to “prove” that the area of a rectangle could be constituted by multiplication of its width and length. Rather than challenging something that the students all knew to be true, the teacher (Simon) directed the conversation toward explanation, subtly reorienting the grounds of argument from the particular to the general (e.g., whether this would work all the time). Simon’s emphasis on the general was further illustrated in another episode in which students attempted to determine the area of an irregular blob by transforming its contour to a more familiar form. Although students could see in a case that their strategy in fact also transformed the area, they were not bothered by this refutation (see also Schauble, 1996), a manifestation of an everyday sense of the general, rather than a mathematical sense. Simon and Blume (1996) also encountered the limits of persuasion when students considered justifications of their predictions about the taste of mixtures that were in different ratios. Here students talked past one another, apparently because some thought of the situation as additive and others as multiplicative. Such studies of teaching and learning again emphasize both the role of the teacher in establishing formats of argument consistent with the discipline and the need for enculturation so that students can see the functions of proof, not simple exposure to proof practices.
Reprise of Mathematical Argument
Mathematical argument emphasizes generality and certainty about patterns and is supported by cognitive capacities to representpossibilityandtoreasoncounterfactuallyaboutpossible patterns. These capacities seem to be robustly supported by cultural practices such as pretense and storytelling. Nevertheless, dispositions to construct mathematically sound arguments apparently do not arise spontaneously in traditional schooling or in everyday cultural practices. Mathematical forms such as proof have their genesis in mathematics classrooms that emphasize conjecture, justification, and explanation. These forms of thinking demand high standards of teaching practice because the evidence suggests that although students may be the primary authors of these arguments, it is the teachers who orchestrate them. Classroom dialogue can spawn overlapping epistemologies, as students are oriented toward mathematics as structure and pattern while they simultaneously examine the grounds of knowledge. Ideally, pattern and proof epistemologies co-originatein classrooms because pattern provides the grounds for proof and proof the rationale for pattern. Thus, classroom conversation and dialogue constitute one possible genetic pathway toward the development of proof reasoning skills and an appreciation of the epistemology of generalization. Yet even as we emphasize proposition and language, we are struck with the role played by symbolization and tools in the development of arguments in classrooms and in various guises of mathematical practice. This is not surprising when one considers the central historical role of such symbolizations in the development of mathematics. We turn next to considering a complementary genetic pathway to mathematical knowledge, that of students as writers of mathematics.
Inscriptions Transform Mathematical Thinking and Learning
In this section we explore the invention and appropriation of inscriptions (literal marks on paper or other media, following Latour, 1990) as mediational tools that can transform mathematical activity. This view follows from our emphasis in the previous section on mathematics as a discursive practice in which everyday resources, such as conversation and pretense, provide a genetic pathway for the development of an epistemology of mathematical argument, of literally talking mathematics into being (Sfard, 2000; Sfard & Kieran, 2001). Here we focus on the flip side of the coin, portraying mathematics as a particular kind of written discourse— “a business of making and remaking permanent inscriptions . . . operated upon, transformed, indexed, amalgamated” (Rotman, 1993, p. 25). Rotman distinguished this view from a dualist view of symbol and referent as having independent existence, proposing instead that signifier (inscription) and signified (mathematical idea) are “co-creative and mutually originative” (p. 33). Accordingly, we first describe perspectives that frame inscriptions as mediators of mathematical and scientific activity, with attention to sociocultural accounts of inscription and argument. These accounts of inscription buttress the semiotic approach taken by Rotman (1988, 1993) and set the stage for cognitive studies of inscription. We go on to describe children’s efforts to invent or appropriate inscriptions in everyday contexts such as drawing or problem solving. Collectively, these studies suggest that the growth of representational competence, as reviewed in the previous section, is mirrored by a corresponding competence in the uses of inscription and notation. In other words, the having of ideas and the inscribing of ideas coevolve. Studies of inscriptionally mediated thinking in mathematics indicate that mathematical objects are created as they are inscribed. This perspective calls into question typical accounts in cognitive science, where inscriptions are regarded as simply referring to mathematical objects, rather than constituting them. We conclude this section with the implications of these findings for an emerging arena of dynamic inscriptions, namely, computational media.
Disciplinary Practices of Inscription and Notation
Studies in the sociology of science demonstrate that scientists invent and appropriate inscriptions as part of their everyday practice (Latour, 1987, 1990; Lynch, 1990). Historically, systems of inscription and notation have played important roles in the quantification of natural reality (Crosby, 1997) and are tools for modeling the world on paper (Olson, 1994). DiSessa (2000, p. 19) noted,
Not only can new inscription systems and literacies ease learning, as algebra simplified the proofs of Galileo’s theorems, but they may also rearrange the entire terrain. New principles become fundamental and old ones become obvious. Entirely new terrain becomes accessible, and some old terrain becomes boring.
Visualizing Nature
One implication of this view of scientific practice as the invention and manipulation of the world on paper (or electronic screen) is that even apparently individual acts of perceiving the world, such as classifying colors or trees, are mediated by layers of inscription and anchored to the practices of disciplinary communities (Goodwin, 1994, 1996; Latour, 1986). Goodwin (1994) suggested that inscriptions do not mirror discourse in a discipline but complement it, so that professional practices in mathematics and science use “the distinctive characteristics of the material world to organize phenomena in ways that spoken language cannot—for example, by collecting records of a range of disparate events onto a single visible surface” (p. 611). For example, archaeologists classify a soil sample by layering inscriptions, field practices, and particular forms of talk to render a professional judgment (Goodwin, 2000). Instead of merely looking, archaeologists juxtapose the soil sample with an inscription (the Munsell color chart) that arranges color gradations into an ordered grid, and they spray water on the soil to create a consistent viewing environment. These practices format discussion of the appropriate classification and illustrate the moment-to-moment embedding of inscription within particular practices.
Repurposing Inscription
Inscriptions in scientific practice are not necessarily stable. Kaiser (2000) examined the long-term history of physicists’ use of Feynman diagrams. Initially, these diagrams were invented to streamline, and make visible, computationally intensive components of quantum field theory. They drew heavily on a previous inscription, Minkowski’s space-time diagrams, which lent an interpretation of Feynman diagrams as literal trajectories of particles through space and time. Of course, physicists knew perfectly well that the trajectories so described did not correspond to reality, but that interpretation was a convenient fiction, much in the manner in which physicists often talk about subatomic particles as if they were macroscopic objects (e.g., Ochs, Jacoby, & Gonzales, 1994; Ochs, Gonzales, & Jacoby, 1996). Over time, the theory for which Feynman developed his diagrams was displaced, and a competing inscription tuned to the new theory, dual diagrams, was introduced. Yet despite its computational advantages, the new inscription (dual diagrams) never replaced the Feynman diagram. Kaiser (2000) suggested that the reason was that the Fenyman diagrams had visual elements in common with the inscriptions of paths in bubble chambers, and this correspondence again had an appeal to realism:
Unlike the dual diagrams, Feynman diagrams could evoke, in an unspoken way, the scatterings and propagation of real particles, with “realist” associations for those physicists already awash in a steady stream of bubble chamber photographs, in ways that the dual diagrams simply did not encourage. (Kaiser, 2000, pp. 76–77)
Hence, scientific practices of inscription are saturated in some ways with epistemic stances toward the world and thus cannot be understood outside of these views.
Inscription and Argument
Nevertheless, Latour (1990) suggested that systems of inscription, whether they are about archaeology or particle physics, share properties that make them especially well suited for mobilizing cognitive and social resources in service of argument. His candidates include (a) the literal mobility and immutability of inscriptions, which tend to obliterate barriers of space and time and fix change, effectively freezing and preserving it so that it can serve as the object of reflection; (b) the scalability and reproducibility of inscriptions, which guarantee economy even as they preserve the configuration of relations among elements of the system represented by the inscription; and (c) the potential for recombination and superimposition of inscriptions, which generate structures and patterns that might not otherwise be visible or even conceivable. Lynch (1990) reminded us, too, that inscriptions not only preserve change, but edit it as well: Inscriptions reduce and enhance information. In the next section we turn toward studies of the development of children as inscribers, with an eye toward continuities (and some discontinuities) between inscriptions in scientific and everyday activity.
The Development of Inscriptions as Tools for Thought
Children’s inscriptions range from commonplace drawings (e.g., Goodnow, 1977) to symbolic relations among maps, scale models, and pictures and their referents (e.g., DeLoache, 1987) to notational systems for music (e.g., Cohen, 1985), number(e.g.,Munn,1998),andtheshapeofspace(Newcombe & Huttenlocher, 2000). These inscriptional skills influence each other so that collectively children develop an ensemble of inscriptional forms (Lee & Karmiloff-Smith, 1996).As a consequence, by the age of 4 years children typically appreciate distinctions among alphabetical, numerical, and other forms of inscription (Karmiloff-Smith, 1992).
Somewhat surprisingly, children invent inscriptions as tools for a comparatively wide range of circumstances and goals. Cohen (1985) examined how children ranging in age from 5 to 11 years created inscriptions of musical tunes they first heard, and then attempted to play with their invented scores. She found that children produced a remarkable diversity of inscriptions that did the job. Moreover, a substantial majority of the 8- to 11-year-olds created the same inscriptions for encoding and decoding. Their inscriptions adhered to one-to-one mapping rules so that, for example, symbols consistently had one meaning (e.g., a triangle might denote a brief duration) and each meaning (e.g., a particular note) was represented by only one symbol. Both of these properties are hallmarks of conventional systems of notation (e.g., Goodman, 1976). Other studies of cognitive development focus on children’s developing understandings and uses of inscription for solving puzzle-like problems.
Karmiloff-Smith (1979) had children (7–12 years) create an inscriptional system that could be used as an external memory for driving (with a toy ambulance) a route with a series of bifurcations. Children invented a wide range of adequate mnemonic marks, including maps, routes (e.g., R and L to indicate directions), arrows, weighted lines, and the like. Often, children changed their inscriptions during the course of the task, suggesting that children transform inscriptions in response to local variation in problem solving. All of their revisions in this task involved making information that was implicit, albeit economically rendered, explicit (e.g., adding an additional mark to indicate an acceptable or unacceptable branch), even though the less redundant systems appeared adequate to the task. Karmiloff-Smith (1992) suggested that these inscriptional changes reflected change in internal representations of the task. An alternative interpretation is that children became increasingly aware of the functions of inscription, so that in this task with large memory demands, changes to a more redundant system of encoding provided multiple cues and so lightened the burden of decoding—a tradeoff between encoding and decoding demands.
Communicative considerations are paramount in other studies of children’s revisions of inscriptions. For example, both younger (8–9 years) and older (10–11 years) children adjusted inscriptions designed as aides for others (a peer or a younger child) to solve a puzzle problem in light of the age of the addressee (Lee, Karmiloff-Smith, Cameron, & Dodsworth, 1998). Compared with adults, younger children were more likely to choose minimal over redundant inscriptions for the younger addressee, whereas the older children were equally likely to chose either inscription. Overall, there was a trend for older children to assume that younger addressees might benefit from redundancy.
In a series of studies with older children (sixth grade through high school), diSessa and his colleagues (diSessa, in press; diSessa, Hammer, Sherin, & Kolpakowski, 1991) investigated what students know about inscriptions in a general sense. They found that like younger children, older children and adolescents invented rich arrays of inscriptions tuned to particular goals and purposes. Furthermore, participants’ inventions were guided by criteria such as parsimony, economy, compactness (spatially compact inscriptions were preferred), and objectivity (inscriptions sensitive to audience, so that personal and idiosyncratic features were often suppressed).
Collectively, studies of children’s development suggest an emerging sense of the uses and skills of inscription across a comparatively wide range of phenomena. Invented inscriptions are generative and responsive to aspects of situation. They are also effective: They work to achieve the goal at hand. Both younger and older children adapt features of inscriptions in light of the intended audience, suggesting an early distinction between idiosyncratic and public functions of inscription. Children’s invention and use of inscriptions are increasingly governed by an emerging meta-knowledge about inscriptions, which diSessa et al. (1991) termed metarepresentational competence. Such capacities ground the deployment of inscriptions for mathematical activity, although we shall suggest (much as we did for argument) that if mathematics and inscription are to emerge in coordination, careful attention must be paid to the design of mathematics education.
Inscriptions as Mediators of Mathematical Activity and Reasoning
Mathematical inscriptions mediate mathematical activity and reasoning. This position contrasts with inscriptions as mere recordsofpreviousthoughtorassimpleconveniencesforsyntactic manipulation. In this section we trace the ontogenesis of this form of mediated activity, beginning with children’s early experiences with parents and culminating with classrooms where inscriptions are recruited to create and sustain mathematical arguments.
Early Development
Van Oers (2000, in press) claimed that early parent-child interactions and play in preschool with counting games set the stage for fixing and selecting portions of counting via inscription. In his account, when a child counts, parents have the opportunity to interpret that activity as referring to cardinality instead of mere succession. For example, as a child completes his or her count, perhaps a parent holds up fingers to signify the quantity and repeats the last word in the counting sequence (e.g., 3 of 1, 2, 3). This act of inscription, although perhaps crudely expressed as finger tallies, curtails the activity of counting and signifies its cardinality. As suggested by Latour (1990), the word or tally (or numeral) can be transported across different situations, such as three candies or three cars, so number becomes mobile as it is recruited to situations of “how many.”
Pursuing the role of inscription in developing early number sense, Munn (1998) investigated how preschool children’s use of numeric notation might transform their understanding of number. She asked young children to participate in a “secret addition” task. First children saw blocks in containers, and then they wrote a label for the quantity (e.g., with tallies) on the cover of each of four containers. The quantity in one container was covertly increased, and children were asked to discover which of the containers had been incremented. The critical behavior was the child’s search strategy. Some children guessed, and others thought that they had to look in each container and try to recall its previous state. However, many used the numerical labels they had written to check the quantity of a container against its previous state. Munn found that over time, preschoolers were more likely to use their numeric inscriptions in their search for the added block, using inscriptions of quantity to compare past and current quantities. In her view, children’s notations transformed the nature of their activity, signaling an early integration of inscriptions and conceptions of number.
Coconstitution of conceptions of number and inscription may also rely on children’s capacity for analogy. Brizuela (1997) described how a child in kindergarten came to understand positional notation of number by analogy to the use of capital letters in writing. For this child, the 3 in 34 was a “capital number,” signifying by position in a manner reminiscent of signaling the beginning of a sentence with a capital letter.
Microgenetic Studies of Appropriation of Inscription
The cocreation of mathematical thought and inscription is elaborated by microgenetic examination of mathematical activity of individuals in a diverse range of settings. Hall (1990, 1996) investigated the inscriptions generated by algebra problem solvers (ranging from middle school to adult participants, including teachers) during the course of solution. He suggested that the quantitative inferences made by solvers were obtained within representational niches defined by interaction among varied forms of inscription (e.g., algebraic expressions, diagrams, tables) and narratives, not as a simple result of parsing strings of expressions. These niches or material designs helped participants visualize relations among quantities and stabilized otherwise shifting frames of reference.
Coevolution of inscription and thinking was also prominent in Meira’s (1995, in press) investigations of (middle school) student thinking about linear functions that describe physical devices, such as winches or springs. His analysis focused on student construction and use of a table of values to describe relations among variables such as the turns of a winch and the distance an object travels. As pairs of students solved problems, Meira (1995) noted shifting signification, reminiscent of the role of the Feynman diagrams, in that marks initially representing weight shifted to represent distance. He also observed several different representational niches (e.g., transforming a group of inscriptions into a single unit and then using that unit in subsequent calculation), a clear dependence of problem-solving strategies on qualities of the number tables, and a lifting away from the physical devices to operations in the world of the inscriptions—a way of learning to see the world through inscriptions.
Izsak (2000) found that pairs of eighth-grade students experimented with different possibilities for algebraic expressions as they explored the alignment between computations on paper and the behavior of the winch featured in the Meira (1995) study. Pairs also negotiated shifting signification between symbols and aspects of device behavior, suggesting that interplay between mathematical expression and qualities of the world may constitute one genetic pathway for mediating mathematical thinking via inscriptions. (We pick this theme up again in the section on mathematical modeling.)
In their studies of student appropriation of graphical displays, Nemirovsky and his colleagues (Nemirovsky & Monk, 2000; Nemirovsky, Tierney, & Wright, 1998) suggested that learning to see the world through systems of inscription is more accurately described as a fusion between signifiers and signified. In their view, coming to interpret an inscription mathematically often involves treating the signifiers and the signified as undifferentiated, even though one knows very well that they can be treated distinctly (the roots of these capabilities are likely found in pretense and possibility, as we described previously). In their studies of students’ attempts to interpret graphical displays of physical motion, they recounted an instance of teacher scaffolding by using “these” to refer simultaneously to lines on a graph, objects (toy bears), and a narrative in which the bears were nearing the finish of a race. This referential ambiguity helped the student create an interpretation of the inscription that was more consistent with disciplinary practice as she sorted out the relations among inscription, object, and the ongoing narrative that anchored use of the inscription to a time course of events.
According to Stevens and Hall (1998), mathematical learning mediated by inscription is tantamount to disciplining one’s perception: coming to see the inscription as a mathematical marking consistent with disciplinary interpretations, rather than as a material object consistent with everyday interpretations. That such a specialized form of perception is required is evident in the confusions that even older students have about forms of notation like the graph of a linear function. For example, a student’s interpretation of slope in a case study conducted by Schoenfeld, Smith, and Arcavi (1993) included a conception of the line as varying with slope, yintercept, and x-intercept. The result was that the student’s conception of slope was not stable across contexts of use.
Stevens and Hall (1998) traced the interventions of a tutor who helped an eighth-grade student working on similar problems of interpretation of graphical displays. Their analysis focused on the tutoring moves that helped reduce the student’s dependence on a literal grid representing Cartesian coordinates. Some of the teacher’s assistance included literal occlusion of grid, a move designed to promote disciplinary understanding by literally short-circuiting the student’s reliance on the grid in order to promote a disciplinary focus on ratio of change to describe the line. Moschkovich (1996) examined how pairs of ninth-grade students came to discipline their own perceptions by coordinating talk, gestures, and inscriptions of slope and intercept. Inscriptions helped orient students toward a shared object of reference, and the use of everyday metaphors such as hills and steepness grounded this joint focus of conversation. Ultimately, however, the relative ambiguity of these everyday metaphors instigated (for some pairs) a more disciplined interpretation because meanings for these terms proved ambiguous in the context of conversation. However, not all pairs of students evolved toward disciplinary-centered interpretation, again suggesting the need for instructional support.
Studies of Inscription in Classrooms Designed to Support Invention and Appropriation
Some research provides glimpses of invention and use of inscription in classrooms where the design of instruction supports students’ invention and appropriation of varying forms of mathematical inscription. These studies are oriented towardacollectivelevelofanalysis(i.e.,treatingtheclassasa unit of analysis) because the premise is that, following Latour (1990), inscriptions mobilize arguments in particular communities. In these studies the community is the mathematics culture of the classroom. Moreover, “a focus on inscriptions requires traditional learning environments to be redesigned in such a way that students can appropriate inscription-related practices and discourses” (Roth & McGinn, 1998, p. 52).
Cobb, Gravemeijer, Yackel, McClain, and Whitenack (1997) traced children’s coordination of units of 10 and 1 in a first grade class. Instruction was designed to situate investigation of these units and unit collections in a context of packaging candies. Arithmetic reasoning was constituted as a “chain of signification” (Walkerdine, 1988) in which unifix cubes first signified a quantity of candies packed in the shop and then this sign (the unifix cubes–candies relation) was incorporated as a signified of various partitions of candies inscribed as pictured collections. At this point the structure of the collection, rather than the original packaging of candy, became the object of thinking. The structure of the collection, in turn, served as the signified of yet another signifier, a notational rendering of collections as, for instance, 3r13c (3 rolls, 13 candies). Cobb et al. (1997) noted that this rendering served as the vehicle by means of which the pictured collections became models of arithmetic reasoning (also see Gravemeijer, Cobb, Bowers, & Whitenack, 2000).
Kemeny (2001) examined the collective dialogic processes during a lesson in which a third-grade teacher helped students construct the mathematical object referred to by the inscription of the Cartesian system. Her analysis underscores the interplay between collective argument and inscription. It also highlights the role of the teacher’s orchestration of conversation and inscription. First, the teacher introduced a new signifier, drawing the axes of the coordinate system on the blackboard, and invited students to consider whether it might be a good tool for thinking about relationships between the sides of similar rectangles. Because these students had a prior history of investigating concepts of ratio via the study of geometric similarity (Lehrer, Strom, & Confrey, in press), the introduction of the signifier (the inscription) created an opportunity for students to create the signified—the Cartesian grid (see Sfard, 2000). Children’s first attempts to generate a signified were based on projecting metaphors of measure. They decided, for example, that the lengths of the axes should be subdivided into equal measures and that this subdivision implied an origin labeled numerically as zero because movement along the axis was a distance, not a count. They debated where this origin should be placed and generated several valid alternatives. At this point, the teacher stepped in to introduce a convention, which students accepted as sensible.
Some students then transported a practice they had generated in previous investigations, superimposing paper models of similar rectangles to observe their growth, to the axes on the blackboard, drawing rectangles that mimicked the paper material. This invited consideration of the axes as a literal support (and raised questions about what to label them), but it also inspired one student to notice a stunning possibility— a rectangle might be represented by one of its vertices. Perhaps there was no need to draw the whole thing! Their teacher promptly seized upon this suggestion, and the students went on to explore its implications. Eventually, they concluded that there could be as many rectangles as they liked, not just the cases initially considered, and that all similar rectangles could be represented and generated as a line through the origin.
Inscription (Cartesian coordinates) and argument (a generalization about similar figures) were co-originated. The inscription did not spring out of thin air, but it became a target of metaphoric projection and extension and was ultimately treated as an object in its own right. The construction of this object invited a format for generalization, the line representing all rectangles, and also an epistemology of pattern. What was true for three or four cases was accepted as true for infinitely many. Over the course of several lessons, students’ inscriptions of similarity as numeric ratio, as algebraic pattern (e.g., the class of similar rectangles described by LS 3 SS, where LS and SS refer to “long side” and
“short side,” respectively), and as a line in the Cartesian system introduced a resonance among inscriptional forms. For example, the sense of pattern generalization could be expressed in three distinctive forms of inscription, yet the equivalence of these forms invited construction of a signified that spanned all three (Lehrer et al., in press).
The lesson analyzed by Kemeny (2001) was anchored in a history of inscription in the classroom (Lehrer, Jacobson, Kemeny, & Strom, 1999; Lehrer & Pritchard, in press). The norms in the classroom included a stance toward adopting inscriptions as tools for thinking and, further, toward assuming that no inscription would be wasted; that is, if students developed a stable (and public) system of mathematical inscription, they could reasonably expect to use it again. One such opportunity was presented to students later in the year when they conducted investigations about the growth of plants. Lehrer, Schauble, Carpenter, and Penner (2000) tracked students’ inscriptions of plant growth during successive phases of inquiry over the course of approximately three months. The investigators found a reflexive relationship between children’s inscriptions of growth and their ideas about growth. Over time, children either invented or appropriated inscriptions that increasingly drew things together by increasing the dimensionality of their models of growth. For example, initial inscriptions were one-dimensional records of height, but these were later supplanted by models of plant volume that incorporated variables of height, width, and depth and that were sequenced chronologically to facilitate test of the conjecture that plant growth was an analogue of geometric growth (which it was not). Inscription and conception of growth were co-originated in Rotman’s (1993) sense.
Notation: A Privileged Inscription
Developmental studies of children’s symbolization, microgenetic studies of individuals’efforts to appropriate inscription, and collective studies of classrooms where inscriptions are recruited to argument describe a complementary genetic pathway for the development of mathematical reasoning: the interactive constitution of inscription and mathematical objects. These studies also reveal the cognitive and social virtues of privileging notations among inscriptions.
Goodman (1976) suggested heuristic principles to distinguish notational systems from other systems of inscription. The principles govern relations among inscriptions (signifiers–literal markings), objects (signified), character classes (equivalent inscriptions, such as different renderings of the numeral 7), and compliance classes (equivalent objects, such as dense materials or emotional people). Two principles govern qualities of inscriptions that qualify as notation: (a) syntactic disjointedness, meaning that each inscription belongs to only one character class (e.g., the marking 7 is recognized as a member of a class of numeral 7s, but not numeral 1s), and (b) syntactic differentiation, meaning that one can readily determine the intended referent of each mark (e.g., if one marked quantity with length, then the differences in length corresponding to differences in quantity should be perceived readily).
Two other principles regulate mappings between character classes and compliance classes. The first is that all inscriptions of a character class should have the same compliance class, which Goodman (1976) referred to as a principle of unambiguity. For example, all numeral 7s should refer to the same quantity, even though the quantity might be comprised of seven dogs or seven cats. It follows, then, that character classes should not have overlapping fields of compliance classes—the principle of semantic disjointedness. For example, the numeral 7 and the numeral 8 should refer to different quantities. This requirement rules out natural language’s intersecting categories, such as whale and mammal. Finally, a principle of semantic differentiation indicates that every object represented in the notational scheme should be able to be classified discretely (assigned to a compliance class)—a principle of digitalization of even analog qualities. For example, the quantities6.999and7.001mightbeassignedtothequantity7, either as a matter of practicality or as a matter of necessity before the advent of a decimal notation.
These features of notational systems afford the capacity to treat symbolic expressions as things in themselves, and thus to perform operations on the symbols without regarding what they might refer to. This capacity for symbolically mediated generalization creates a new faculty for mathematical reasoning and argument (Kaput, 1991, 1992; Kaput & Schaffer, in press). For example, the well-formedness of notations makes algorithms possible, transforming ideas into computations (Berlinski, 2000). Notational systems simultaneously provide systematic opportunity for student expression of mathematical ideas, but the same systematicity places fruitful constraints on that expression (Thompson, 1992).
We have seen, too, how notations transform mathematical experiences genetically, both over the life span (from early childhood to adulthood) and over the span of growing expertise (from novices to professional practitioners of mathematics and science). Consider, for example, the van Oers (2000, in press) account of parental scaffolding to notate children’s counting. This marking objectifies counting activity so that it becomes more visible and entity-like. The use of a symbolic system for number foregrounds the quantity that results from the activity of counting and backgrounds the counting act itself. This separation of activity (counting) from its product (quantity) sets the stage for making quantity a substrate for further mathematical activities, such as counts of quantities as exemplified in the Cobb et al. (1997 )study of first graders. Microgenetic studies like those of Hall (1990) and Meira (1995) suggest that inscriptions tend to drift over time and use toward notations that stabilize interactions among participants. The classroom studies by Kemeny (2001) and Lehrer et al. (2000) also suggest a press toward notation as a means of fixing, selecting, and composing mathematical objectsastoolsforargument.Thesestudies,however,concentrate largely on the world on paper, so in the next section we address the implications of electronic technologies for bootstrapping the reflexive relation between conception and inscription.
Dynamic Notations
The chief effect of electronic technologies is the corresponding development of new kinds of notational systems, often described as dynamic (Kaput, 1992). The manifestations of electronically mediated notations are diverse, but what they share in common is an expression of mathematics as computation (Noss & Hoyles, 1996). DiSessa (2000) suggested that computation is a new form of mathematical literacy, concluding that computation, especially programming, “turns analysis into experience and allows a connection between analytic forms and their experiential implications” (p. 34). Moreover, simulating experience is a pathway for building students’understanding, yet it is also integral to the professional practices of scientists and engineers.
Sherin (2001) explored the implications of replacing algebraic notation with programming for physics instruction. Here again, notations did not simply describe experience for students, but rather reflexively constituted it. Programming expressions of motion afforded more ready expression of time-varying situations. This instigated a corresponding shift in conception from an algebraically guided physics of balance and equilibrium to a physics of process and cause.
Resnick (1994) pointed out that introducing students to parallel programming (e.g., multiple screen “turtles”) provides an opportunity to develop mathematical descriptions at multiple levels and to understand how levels interact. The programming language provides an avenue for decentralized thinking. Wilensky and Resnick (1999) noted the difficulties that people have in comprehending levels of phenomena such as traffic jams. At one level, traffic jams result from cars moving forward, but the interactions among cars create jams that proliferate backward. This effect seems at first glance to violate common sense, so it is hard for people to comprehend, but dynamic notations such as parallel programming place new tools in the hands of students for thinking about relations between local agents and aggregate levels of description. Our (much) abbreviated tour of dynamic notations clearly indicates that this form of inscription affords new opportunities to coconstitute mathematical thought and writing. In the sections that follow, we revisit this theme in the realms of geometry measurement and mathematical modeling.
Geometry and Measurement
Geometry is a spatial mathematics that has its roots in antiquity yet continues to evolve in the present, as witnessed by continuing concern with computer-generated experiments in visualization. Although common school experiences of geometry emphasize the construction and proof schemes of the ancient Greeks, the scope of geometry is far wider, ranging from consideration of fundamental qualities of space such as shape and dimension (e.g., Banchoff, 1990; Senechal, 1990) to the very fabric of artistic design, commercial craft, and models of natural processes (e.g., Stewart, 1998). Consider, for example, the designs displayed in Figure 15.1. Both were created from the same primary cell (unit) but with different symmetries (the left by a translation, the right by a rotation). Systematic analyses of symmetries of design stimulate both mathematical inquiry (e.g., Schattschneider, 1997; Washburn & Crowe, 1988) and the ongoing practice of crafts such as quilting (e.g. Beyer, 1999).
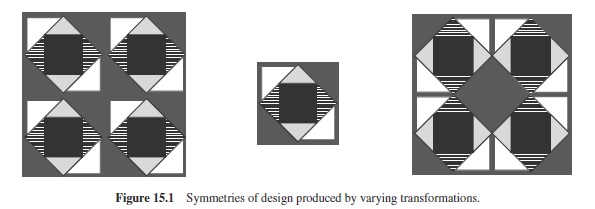
Geometry’s versatility and scope have oriented us to survey a range of studies that demonstrate the potential role of geometry in a general mathematics education (Goldenberg, Cuoco, & Mark, 1998; Gravemeijer, 1998). Our chief emphasis is on studies of the growth and development of spatial reasoning in contexts designed to support development (principally, schools). We first consider studies of children’s unfolding understanding of the measure of space. Although measurement is (now) traditionally separated from geometry education, we argue for its reinstatement on two grounds. First, measuring a quality of a space invokes consideration of its nature. For example, although measure of dimension seems transparent, the dimension of fractal images in not obvious, and consideration of their measure leads one toward more fundamental ideas about their construction (e.g., Devaney, 1998). Second, measurement is inherently approximate so that it constitutes a bridge to related forms of mathematics, such as distribution and reasoning about variation. Third, practices of measurement span multiple realms of endeavor, especially the quantification of physical reality (Crosby, 1997). Even apparently simple acts, such as matching the color of a sample of dirt to an existing classification scheme, are in fact embedded within systems of inscription and practice, so that measurement is a window to the interplay between imagined qualities of the world and the practical grasp of these qualities (Goodwin, 2000). Consequently, our review focuses on research that helps us understand the kinds of thinking at the heart of the interplay between this imaginative leap (i.e., an imagined quality of space) and practical grasp (e.g., its measure).
After completing our review of measure, we consider how inquiry about shape and form frames developing types of arguments, especially proof and related “habits of mind” (Goldenberg et al., 1998). Here we focus on the role of dynamic notational systems, embodied (currently) as software tools such as Logo (Papert, 1980) and the Geometer’s Sketchpad (Jackiw, 1995), because these spotlight the role of dynamic notation in the development of mathematical reasoning and argument about space.
The Measure of Space
In the sections that follow, we review investigations of children’s reasoning about measure. We focus primarily on studies of linear measure to illuminate the interactive roles of inscription and developing conceptions of space because these studies encapsulate many of the findings, issues, and approaches that emerge in investigations of other dimensions and qualities of space, such as area, volume, and angle (see Lehrer, 2002; Lehrer, Jaslow & Curtis, in press, for more extensive review of the latter). We include studies from multiple perspectives. Studies of cognitive development typically compare children at different ages (cross-sectional) or follow the same children for a period of time (longitudinal) to observe transitions in thinking, typically about units of measure. These studies provide glimpses of children’s thinking under conditions of activity and learning that are typically found in the culture. They follow from the tradition first established by Piaget and his colleagues (e.g., Piaget, Inhelder, & Szeminska, 1960). In contrast, design studies modify the learning environment and then investigate the effects of these modifications (Brown, 1992; Cobb, 2001). These studies are often conducted from sociocultural perspectives with attendant attention to forms of inscription and notation and to forms of classroom talk that seem important to help learning to push development in the manner first articulated by Vygotsky (1978).
Mental Representation of Distance
Piaget et al. (1960) proposed that to obtain a measure of length, one must subdivide a distance and translate the subdivision. Thus, n iterations of a unit represent a distance of n units. Because distance is not a topological feature, Piaget et al. (1960) proposed that children may fail to understand that translation does not affect distance (i.e., that simple motion of a length does not change its measure), a symptom in Piaget’s view of topological primacy in children’s representations of space. For example, preschool children often assert that objects become closer together when they are occluded. Piaget et al. (1960) believed that this assertion revealed children’s use of a topological representation that would preserve features such as continuity between points but not (necessarily) distance because occlusion disrupts the topological property of continuity.
A series of experiments conducted by Miller and Baillargeon (1990) suggested instead that children’s assertions reflected their relative perceptions of occluded and unoccluded distances. Children from 3 to 6 years of age proposed wooden lengths that would span a distance between two endpoints of a bridge. The distance was then partially occluded. Although children often reported that the occluded endpoints were closer together, they also asserted that the length of the stick that “just fit” between them was unaffected. This lack of correspondence between what children said and what they did refuted the topological hypothesis, indicating instead that children’s responses were guided by appearances, not mental representations of distance governed by continuity of points. Research solidly refutes Piaget’s equating of the historic structuring of geometries (e.g., progressing from Euclidean to topological) to changes over the life span in ways of mentally representing space (e.g., Darke, 1982). For example, more contemporary research demonstrates that infants (and rats) encode (Euclidean) metric information (see Newcombe & Huttenlocher, 2000). Although it then seems reasonable to assume an implicit metric representation of distance, Piaget’s core agenda of documenting transitions in children’s constructions of invariants about units of measure has proven fruitful.
Developing Conceptions of Unit
Children’s first understandings of length measure often involve direct comparison of objects (Lindquist, 1989; Piaget et al., 1960). Congruent objects have equal lengths, and congruency is readily tested when objects can be superimposed or juxtaposed. Young children (first grade) also typically understand that the length of two objects can be compared by representing them with a string or paper strip (Hiebert, 1981a, 1981b). This use of representational means likely draws on experiences of objects “standing for” others in early childhood, as we described previously. First graders (6- and 7-year-olds) can use given units to find the length of different objects, and they associate higher counts with longer objects (Hiebert, 1981a, 1981b; 1984). Most young children (first and second graders) even understand that, given the same length to measure, counts of smaller units will be larger than counts of larger units (Carpenter & Lewis, 1976).
Lehrer, Jenkins, and Osana (1998) conducted a longitudinal investigation of children’s conceptions of measurement in the primary grades (a mixed age cohort of first-, second-, and third-grade children were followed for three years). They found that children in the primary grades (Grades 1–3, ages 6–8) may understand qualities of measure like the inverse relation between counts and size of units yet fail to appreciate other constituents of length measure, like the function of identical units or the operation of iteration of unit. Children in this longitudinal investigation often did not create units of equal size for length measure (Miller, 1984), and even when provided equal units, first and second graders typically did not understand their purposes, so they freely mixed, for example, inches and centimeters, counting all to measure a length.
For these students, measure was not significantly differentiated from counting (Hatano & Ito, 1965). Thus, younger students in the Lehrer, Jenkins, et al. (1998) study often imposed their thumbs, pencil erasers, or other invented units on a length, counting each but failing to attend to inconsistencies among these invented units (and often mixing their inventions with other units). Even given identical units, significant minorities of young children failed to iterate spontaneously units of measure when they ran out of units, despite demonstrating procedural competence with rulers (Hatano & Ito, 1965). For example, given 8 units and a 12-unit length, some primary-grade children in the longitudinal study sequenced all 8 units end to end and then decided that they could not proceed further. They could not conceive of how one could reuse any of the eight units, indicating that they had not mentally subdivided the remaining space into unit partitions.
Children often coordinate some of the components of iteration (e.g., use of units of constant size, repeated application) but not others, such as tiling (filling the distance with units). Hence, children in the primary grades occasionally leave spaces between identical units even as they repeatedly use a single unit to measure a length (Lehrer, 2002). The components of unit iterations that children employ appear highly idiosyncratic, most likely reflecting individual differences in histories of learning (Lehrer, Jenkins, et al., 1998).
Developing Conceptions of Scale
Measure of length involves not only the construction of unit but also the coordination of these units into scales. Scales reduce measurement to perception so that the measure of length can be read as a point on that scale. However, only a minority of young children understand that any point on a scale of length can serve as the starting point, and even a significant minority of older children (e.g., fifth graders) respond to nonzero origins by simply reading off whatever number on a ruler aligns with the end of the object (Lehrer, Jenkins, et al., 1998).
Many children throughout schooling begin measuring with one rather than with zero (Ellis, Siegler, & Van Voorhis, 2000). Starting a measure with one rather than zero may reflect what Lakoff and Nunez (2000) referred to as metaphoric blend. One everyday metaphor for measure is that of the measuring stick, where physical segments such as body parts (e.g., hands) are iterated and the basic unit is one stick. Another everyday metaphor is that of motion along a path, corresponding to children’s experiences of walking (Lakoff & Nunez, 2000). Measure of a distance is then a blend of motion and measuring-stick metaphors, which may lead to mismappings between the 1 count of unit sticks and 0 as the origin of the path distance (Lehrer et al., in press). The difficulties entailed by this metaphoric blend are often most evident when children need to develop measures that involve partitions of units. For example, Lehrer, Jacobson, Kemeny, and Strom (1999) noted that some second-grade children (7–8 years of age) measured a 2 12-unit strip of paper as 3 12 units by counting, “1, 2, [pause], 3 [pause], 3 12.” They explained that the 3 referred to the third unit counted, but “there’s only a 12,” so in effect the last unit was represented twice, first as a count of unit and then as a partition of a unit. Yet these same children could readily coordinate different starting and ending points for integers (e.g., starting at 3 and ending at 7 was understood to yield the same measure as starting at 1 and ending at 5).
Design Studies
Design studies focus on establishing developmental trajectories for children’s conceptions of linear measure in contexts designed to promote children’s use of inscription and tools. These tools and inscriptions are typically objects of conversation in classrooms, recruited to resolve contested claims about comparative lengths of objects or about reasonable estimates of an object’s length. Hence, these studies are representative of contexts in which conversation, inscription, and tool use are typically interwoven.
Inscriptions and Tools Mediate Development of Conceptions of Measure
Choices of tools often have consequences for children’s conceptions of length (Nunes, Light, & Mason, 1993). Clements, Battista, and Sarama (1998) reported that using computer tools that mediated children’s experience of unit and iteration helped children mentally restructure lengths into units. Third graders (9-year-olds) created paths on a computer screen with the Logo programming language. Many activities focused on composing and decomposing lengths, which, in combination with the tool, encouraged students to privilege some segments and their associated command (e.g., forward 10) as units. Subsequently, children found unknown distances by iterations of these units. For example, one student found a length of 40 turtle units by iterating 10 turtle units. Students in this and related investigations apparently developed conceptual rulers to project onto unmarked segments (Clements, Battista, Sarama, Swaminathan, & McMillen, 1997). In an investigation conducted by Watt (1998), fifth-grade students employed a children’s computer-aided design tool, kidCAD, to create blueprints of their classroom. At the outset of the investigation, students displayed many of the hallmarks of conceptions of measure that one might expect from the studies of cognitive development. That is, students evidenced tenuous grasp of the zero point of the measurement scale and mixed units of length measure. Here, students’efforts to create consistency between their kidCAD models and their classroom helped make evident the rationale for measurement conventions. These recognitions led to changes in measurement practices and conceptions.
Other studies place a premium on children’s constructions of tools and inscriptions for practical measurements. This form of practical activity facilitates transition from embodied activity of length measure, such as pacing, to symbolizing these activities as “foot strips” and related measurement tools (Lehrer et al., 1999; McClain, Cobb, Gravemeijer, & Estes, 1999). By constructing tools and inscribing units of measure, children have the opportunity to discover, with guidance, how scales are constructed. For example, children often puzzle about the meaning of the marks on rulers, and the functions of these marks become evident to children as they attempt to inscribe units and parts of units on their foot strips (Lehrer et al., 1999). Moreover, when all students do not employ the same unit of measure, the resulting mismatches in the measure of any object’s length spurs the need for a conventional unit (Lehrer et al., in press). These mismatches highlight that measurement is not purely a cognitive act. It also relies on perceiving the social utility of conventional units and the communicative function served by common methods of measure.
Tools Enhance the Visibility of Children’s Thinking for Teachers
The construction of tools also makes children’s thinking more visible to teachers, who can then transform instruction as needed (Lehrer et al., in press). For example, Figure 15.2 displays a facsimile of a foot-strip tape measure designed by a third-grade student, Ike, who indicated that the measure of the ruler’s length was 4 because 4 footprint units fit on the tape. Some components of iteration of unit are salient; the units are all alike, and they are sequenced. On the other hand, the process to be repeated appears to be a count, rather than a measure, as indicated by the lack of tiling (space filling) of the units. Construction of this tool mediated this student’s understanding of unit, helping make salient some qualities of unit. As we noted previously, these qualities of selection and lifting away from the plane of activity are commonplace features of notational systems. Other qualities that were evident in this student’s paces (when he walked a distance, he placed his feet heel to toe) remained submerged in activity. Hence, in this classroom, creation of the tool provided a discursive opening for the teacher and for other students who disagreed with Ike’s production and who suggested that perhaps the “spaces mattered.”
Splitting and Rational Number
Measurement can serve as a base metaphor for number. Confrey (1995; Confrey & Smith, 1995) suggested an interpenetration between measure and conceptions of number via splitting. Splitting refers to repeated partitions of a unit to produce multiple similar forms in direct ratio to the splitting factor. For example, halving produces ratios of 1 : 2. Rather than simply split paper strips as an activity for its own sake, measurement provides a rationale for splitting. Consequently, in a classroom study Lehrer et al. (1999) observed secondgrade children repeatedly halving unit lengths as they designed rulers. The need for these partitions of unit arose as children attempted to measure lengths of objects that could not be expressed as whole numbers.
Most children folded their unit (represented as a length of paper strip) in half and then repeated this process to create fourths, eighths, and even sixty fourths. These partitions were then employed in children’s rulers, and children noticed that theycouldincreasetheprecisionofmeasure.Eventually,these actions helped children develop operator conceptions of rational numbers, such as 1 ∕ 2 х 1 ∕ 2 х 1 ∕ 2 = 1 ∕ 8, and so on. Similarly, division concepts of rational numbers were promoted by classroom attention to problems involving exchanges among units of measure for a fixed length. For example, if one Stephanie (unit) is one-half of a Carmen (unit) and a board is 4 Carmens long, what is its measure in Stephanies? The visual relations among paper-strip models of these units helped children differentiate between “one half of” and “divided by one half.” Moss and Case (1999) also featured splitting of linear measurement units as a means to help students develop concepts of rational numbers. Their work with fourth-grade students indicated that measure and splitting, coupled with an emphasis on equivalence among different notations of rational number, helped students develop understanding of proportionality and, correlatively, of rational numbers.
Measure and Modeling as a Gateway to Form
Classroom studies point to ways of melding linear measure and the study of form in the elementary grades in ways that recall their historical codevelopment. Children in Elizabeth Penner’s first- and second-grade classes searched for forms (e.g., lines, triangles, squares) that would model the configuration of players in a fair game of tag (Penner & Lehrer, 2000). Attempts to inscribe the shape of fairness initiated cycles of exploration involving length measure and properties related to length in each form (e.g., distances from the sides of a square to the center). Eventually, children decided that circles were the fairest of all forms because the locus of points defining a circle was equidistant from its center. This insight was achieved by emerging conceptions of units of linear measure (e.g., children created foot strips and other tools to represent their paces) and by employing these understandings to explore properties of shape and form. For example, children were surprised to find that the distance between the center of a square and a side varied with the path chosen. Diagonal paths were longer than those that were perpendicular to a side, so they concluded that square configurations were not fair, despite the congruence of their sides.
Children in Carmen Curtis’s third-grade class investigated plant growth and modeled changes in their canopy as a series of cylinders. Developing the model posed a new challenge in mathematics, namely, grasping the correspondence between a measure of “width” (the diameter of the base of the cylinder) and its circumference. In other words, children could readily measure the width but then had to figure out how diameter could be used to find circumference.This challenge instigated mathematical investigation, one that culminated in an approximation of the relation between circumference and diameter as “about 3 15.” So, in the course of modeling nature, children developed a conjecture about the relationship between properties of a circle. Of course, their investigations did not end here, because having convinced themselves and others about the validity of their model of the canopy of the plant, they next had to concern themselves with how to measure its volume (Lehrer et al., in press). In sum, tight couplings between space and measure in these modeling applications are reminiscent of Piaget’s investigations but acknowledge that these linkages are the object of instructional design, instead of regarding them as preexisting qualities of mind.
Measure and Argument
In some classrooms measures are recruited in service of argument. For example, in one of the second-grade classrooms referred to previously (Lehrer et al., 1999), children saw paper models of three different rectangles and were asked to consider which covered the most space on the blackboard. The rectangles all had the same area but were of different dimension (1 12, 2 6, 3 4 units). The rectangles were not marked in any way, nor were any tools provided. Children’s initial claims were based on mere appearance. Some thought that the “fat” rectangles (i.e., the 3 4) must cover the most space, others that the “long” (i.e., 1 12) rectangles must. These contested claims set the stage for the teacher’s orchestration of argument: How could these claims be resolved? Strom et al. (2001) analyzed the semantic structure of the resulting classroom conversation and rendered its topology as a directed graph. The nodes of the graph consisted of various senses of area as children conceived it (e.g., as space covered, as composed of units), as enacted (e.g., procedures to partition and reallot areas, procedures that privileged certain partitions as units), and as historically situated (e.g., children’s senses of this situation as related to others that they had previously encountered). The analysis highlighted the interplay among these forms of knowledge— an interplay characteristic also of professional practice (e.g., Rotman, 1988)—and illustrated that the genetic trajectories of conceptual, procedural, and historical knowledge were firmly bound, not distinct. Moreover, a pivotal role was played by notating the unit-of-area measure, a process that afforded mobility and consequent widespread deployment of unit in service of argument. That is, once the unit-of-area measure assumed consensual status as a legitimate tool in the classroom, it was used literally to mark off segments of area on the three rectangles, eventually establishing that regardless of appearance, each covered 12 square units of space: All three rectangles covered the same space. Of course, the argument constructed by children was orchestrated by the teacher, who animated certain students’ arguments, juxtaposed temporally distant forms of reasoning, and reminded students of norms of argument and justification throughout the lesson.
Estimation and Error
Much of the research about measurement explores precision and error of measure in relation to mental estimation (Hildreth, 1983; Joram, Subrahmanyam, & Gelman, 1998). To estimate a length, students at all ages typically employ the strategy of mentally iterating standard units (e.g., imagining lining up a ruler with an object). In their review of a number of instructional studies, Joram et al. (1998) suggested that students often develop brittle strategies closely tied to the original context of estimation. Joram et al. recommended that instruction should focus on children’s development of reference points (e.g., landmarks) and on helping children establish reference points and units along a mental number line. It is likely that mental estimation would also be improved with more attention to the nature of unit, as suggested by many of the classroom studies reviewed previously. However, Forrester and Pike (1998) indicated that in some classrooms, estimation is treated dialogically as distinct from measurement. Employing conversation analysis, they examined the discursive status of measurement and estimation in two fifth-grade classrooms. Teachers formatted estimation as an activity that preceded measure and as one characterized by a lack of precision. In contrast, measurement was associated with real length (i.e., perimeter) and the use of a ruler. The consequence of this formatting was that students who employed nonstandard units to estimate, such as their fingers, could not conceive of any way in which the use of such units might be considered as measure. In short, treating estimation and measurement as discursively distinct resulted in a corresponding conceptual division between them.
In contrast, Kerr and Lester (1986) underscored a fusion between measurement and estimation. They suggested that instruction in measure should routinely encompass considerations of sources of error, especially (a) the assumptions (e.g., the model) about the object to be measured, (b) choice of measuring instrument, and (c) how the instrument is used (e.g., method variation). Historically, the recognition of error was troubling to scientists. For example, Porter (1986) documented the struggles in astronomy to come to grips with variability in the measures of interstellar distances. Varelas (1997) examined how third- and fourth-grade students made sense of the variability of repeated trials. Many children apparently did not conceptualize the differences among repeated observations as error and often suggested that fewer trials might be preferable to more. In other words, their solution was to sidestep the problem by avoiding the production of troubling variability. Their conceptions seemed bound with relatively diffuse conceptions of representative values of a set of repeated trials. In a related study, Lehrer et al. (2000) found that with explicit attention to ways of ordering and structuring trial-to-trial variability, second-grade children made sense of trial-to-trial variation by suggesting representative (“typical”) values of sets of trials. Choices of typical values included “middle numbers” (i.e., medians) and modes, with a distinct preference for the latter. In contexts where the distinction between signal and noise was more evident, as in repeated measures of mass and volume of objects, fifth-grade students readily proposed variations of trimmed means as estimates of “real” weights and volumes (Lehrer, Schauble, Strom, & Pligge, 2001).
Petrosino, Lehrer, and Schauble (in press) further investigated children’s ideas about sources and representations of measurement error. In a classroom study with fourth graders, children’s conceptions of error were mediated by the introduction of concepts of distribution. Students readily conceptualized the center of a distribution of measures of the height of the school’s flagpole as an estimate of its real height. Furthermore, indicators of variability were related to sources of error, such as individual differences and differences in tools used to measure height. Hence, students in this fourth-grade classroom came to understand that errors in measure might be random, yet still evidence a structure that could be predicted by information about sources of error, such as instrumentation. Konold and Pollatsek (in press) suggested that contexts of repeated measures like those just described offer significant advantages for assisting students to come to see samples of measures as outcomes of processes, and statistics like center and spread as indicators of signal and noise in these processes, respectively.
Collectively, design studies and research in cognitive development suggest several trends. First, children’s initial understandings of the measurement of length are likely grounded in commonplace experiences like walking and commonplace artifacts, like measuring sticks (e.g., rulers). Accordingly, engaging students in inscribing motion and designing tools leads to significant transitions in conceptual development. These transitions exceed those that one might expect from everyday activity and suggest some of the ways in which instructional design and learning can lead children’s development. Second, understanding of length measure emerges as children coordinate conceptual constituents of the underpinnings of unit, such as subdivision of a length and iteration of these subdivisions, with the underpinnings of scale, such as origin and its numeric representation as zero. These coordinations appear to emerge in pieces, with procedural manipulation of given units to measure a length often preceding fuller understanding of the entailments of these procedures. Constructs of unit are intertwined with those of scale, so that, for example, the correspondence of zero and the origin of a scale likely undergo several transitions.
Third, length measure can serve as a springboard to related forms of mathematics. The continuity of linear measure, coupled with procedures of splitting, appears to offer important resources for the development of rational number concepts. Measure and modeling can also serve as a foundation for children’s conceptions of shape, especially properties of shape. Fourth, measure can be recruited in service of mathematical argument. Such recruitment leads to conceptual change as students grapple with ways of resolving contesting claims by developing and refining their conceptions of unit. Fifth, considering measure as inherently imprecise provides a lead-in to the mathematics of distribution, especially when students are asked to develop accounts (and measures) of the contributions of different sources of error. Measurement processes are a good entry point for distribution because they clarify the contributions both of signal and error to the resulting shape of distribution. Consequently, children can come to see the structure inherent in a random process.
Finally, the need to promote conceptual development about measurement explicitly is acute when one considers that typical beginning university students often exhibit a relatively tenuous grasp of the measure of space. For example, Baturo and Nason (1996) noted that for the majority of a sample of preservice teachers, area measure was tightly bound to recall of formulas, like that used to find the area of a rectangle. Yet none had any idea about the basis of any formula. Most asserted that 128 cm2 were larger than 1 m2 because there were 100 cm in a meter. Many thought that area measure applied only to polygons and confused area with volume when presented with three-dimensional shapes. These fragile conceptions of measure appear similar to those of other preservice teachers as well (e.g., Simon & Blume, 1996, as we described earlier).
Structuring Space
In the preceding section we described how children come to structure space through its measure, assisted by efforts to model and inscribe length. We reprise these themes by turning to studies that describe how children come to structure space through its construction. We focus on dynamic notations afforded by electronic technologies. These electronic technologies loosen the tether of geometry to its euclidean foundation by introducing motion to form, in contrast to the static geometry of the Greeks (Chazan & Yerushalmy, 1998). Motion is inscribed from either local or global perspectives. The former is represented by tools like Logo (Papert, 1980), which approach the tracing of a locus of points through the action of an agent. The agent’s perspective is local because a pattern like a circle or square emerges from a series of movements of the agent, often called a turtle, such as the line segment that results from FD 40 (which traces a path 40 units from the current orientation of the turtle). In contrast, tools like the Geometer’s Sketchpad (Jackiw, 1995) introduce motion from the perspective of the plane so that movement is defined globally by stretching line segments (or entire figures). For example, a construction of a square can be resized by dragging one of its vertices or sides. The resulting dynamic geometry is a new mathematical entity (Goldenberg, Cuoco, & Mark, 1998). So, too, is the geometry afforded by Logo, albeit in a different voice (Abelson & diSessa, 1980).
Potential Affordances of Motion Geometries
Like other innovations in notational systems, agent-based and dynamic geometries afford new ways of thinking about shape and form. Logo (representing agent-based geometries) affords a path perspective to shape and form—one comes to see a figure as a trace of an agent’s (e.g., the turtle’s) motions. It allows procedural specification of figures, thus creating grounds for linking properties of a figure with operations necessary to generate those properties. For example, the three sides and three angles of a triangle correspond to three linear motions (e.g., Forward 70) and three turns (e.g., Right 120) of a turtle. Procedural specification, in turn, affords a distinction between the particular and the general. For instance, any polygon can be defined by the same procedure simply by varying the inputs to that procedure (e.g., the number of sides). Thus, a procedure can simultaneously represent a specific drawn polygon or any polygon. Dynamic geometries (e.g., the Geometer’s Sketchpad) create a clear distinction between the particular and the general in a different way. Drawing allows the creation of particular figures, but construction allows the creation of general figures. The distinction between the two has a practical consequence in dynamic geometry. When dragged (e.g., continuously deforming a shape by pulling on a vertex), the relationships among constituents of drawings change, but the relationships among constituents of constructions do not. The result is that “the diagrams created with geometry construction programs seem poised between the particular and the general. They appear in front of us in all their particularity, but, at the same time, they can be manipulated in ways that indicate the generalities lurking behind the particular” (Chazan & Yerushalmy, 1998, p. 82). So, like Logo, geometry construction environments relax the notational constraint of semantic disjointedness, moving notation in the direction of natural language. The drag mode of dynamic geometries creates multiple examples, and the measurement capabilities of dynamic geometry tools provide a fertile ground for conjecture and experiment. Both Logo and dynamic geometry tools also provide means for individual expression—especially when they are harnessed to design (Harel & Papert, 1991; Lehrer, Guckenberg & Lee, 1988; Shaffer, 1998).
Learning in Motion
What, then, of learning? Do motion geometries create consequential opportunities for pedagogical improvement, or are they simply different? Such questions are fraught with difficulty because media are not neutral, yet their effects are usually bound with the kinds of pedagogical practices that they afford. When these tools for dynamic notation are used in ways that preserve the forms of teaching practice articulated by Schoenfeld (1988; e.g., separating construction and deduction), there seems to be little evidence of any substantive change in student conceptions or epistemologies (Chazan & Yerushalmy, 1998). However, when these tools are coupled with forms of instruction that emphasize conjecture, explanation, and individual expression, the research clearly indicates substantive conceptual change.
Logo Geometry
Perhaps because Logo and its descendants have a longer history, the evidence for learning with Logo spans multiple decades and forms of inquiry. Early studies of learning with Logo were conducted by its founders and featured carefully articulated cases of student investigation of, among other things, conjectures about the invariant sum of the turns (i.e., 360) in the paths of polygons and explorations of the relationships among constituents of shape, such as sides and angles (e.g., Papert, Watt, diSessa, & Weir, 1979). Follow-up studies attempted to articulate relations between teaching and learning with and without Logo tools, and again a subset of this work focused on children’s learning about shape and form.
When students use Logo in environments crafted to invite student investigation and reflection, students (most research was conducted with elementary students) tended to analyze properties of shape and form, such as angle and side, and to develop concepts of definition of classes of forms, as well as relations among classes, such as squares and rectangles (e.g., Clements & Battista, 1989, 1990; Lehrer et al., 1988a; Lehrer, Guckenberg, & Sancilio, 1988b; Noss, 1987; Olive, 1991). Collectively, these studies painted portraits of children’s learning of shape and form that (at the time) appeared unobtainable with conventional tools and instruction. Moreover, children’s responses suggested that their learning followed from their use of Logo tools. For instance, third-grade children often compared forms such as triangles and squares by considering the programs they used to make them: “Well, it’s . . . 3 times 120 here and 4 times 90 here equal 360 and that’s once around” (Lehrer et al., 1988a, p. 548). Moreover, in the Lehrer et al. (1988a) study, independent measures of children’s knowledge of Logo’s turn and move commands and their ability to implement variables (tools for generalization in Logo) correlated substantially with measures of children’s knowledge of angles and of relations among polygons, respectively. Not surprisingly, these effects were stronger when instruction was designed to help students develop knowledge of geometry, rather than simply good programming skills. Lehrer, Randle, and Sancilio (1989) suggested that some of what children were learning with Logo could be attributed to formats of instruction and argument because researchers were often serving as teachers, and most tended to promote conjecture and explanation in their teaching.
Lehrer et al. (1989) worked with groups of fourth-grade children with similar instructional goals and similar emphases on conjecture and explanation, but only some of the students used Logo as a tool. They found no differences between the groups on measures of simple attributes of shape and form, like angle measure or identification of properties like parallelism. However, students using Logo tools learned more about class inclusion relationships among quadrilaterals and were far better at distinguishing necessary and sufficient conditions in the definition of polygons. Moreover, these differences between groups endured beyond the cycle of instruction. Protocol analysis suggested that one likely source of these differences was children’s use of variables to define shapes in ways that allowed them to coconstitute the general (the procedure defined with one or more variables) and the particular (the figure drawn on the screen). Related research with Logo-based microworlds expanded the scope of geometry to transformation and symmetry and to ratio and proportion (Edwards, 1991; see Edwards, 1998; Miller, Lehman, & Koedinger, 1999, for general perspectives on microworlds and learning).
Acontemporary cycle of research featuring Logo as a tool for teaching and learning geometry significantly extends its reach and is best exemplified by the work of Clements, Battista, and Samara (2001), who documented a program of research conducted over the last decade. Teachers in Grades 1 through 6 used a Logo-based curriculum of ambitious scope in which study of shape and form featured cycles of conjecture and explanation. Their results replicated the major findings of previous research but also significantly expanded them to include broader portraits of student learning and development with diverse samples of students (See also Clements, Sarama, Yelland, & Glass, in press). In summary, although the path of research with Logo has hit its share of snags and setbacks, investigations of Logo as a tool for teaching and learning geometry in carefully crafted environments suggest clear support for the claim that it provides a new form of mathematical literacy.
Dynamic Geometries
Research with dynamic geometries, again conducted in environments crafted to support learning, also suggested productive means by which these tools can be harnessed to inform conceptual change. However, our tour of this literature is abbreviated due both to its relative novelty and to the practical limitations of space. Several studies indicate that the distinction between drawings and constructed diagrams exemplified in dynamic geometry tools constitutes a form of instructional capital. Constructions that can be subjected to motion afford systematic experimentation, and this capacity for experimentation can be instructionally focused to a search for an explanation of the invariants observed (Arcavi & Hadas, 2000; de Villiers, 1998; Olive, 1998). Koedinger (1998) proposed an explicit model of instructional support for encouraging generation and refinement of student conjectures, thus changing the grounds of deduction. For example, his model develops a tutoring architecture that supports students’constructions of diagrams and associated experiments. Arcavi and Hadas (2000) described instructional support for use of dynamic geometry tools to model situations, with particular attention to how symbolic expression of function is informed by systematic experimentation. Chazan (1993) found that the use of construction-geometry tools in concert with instruction that supported student conjecturing helped high school students become more aware of distinctions between empirical and deductive forms of argument.
Technologically Assisted Design Tools
Although dynamic geometry tools are most often employed to solve mathematical problems posed by teachers, Shaffer (1997) designed a dynamic geometry construction microworld, Escher’s World, that high school students used for creating artistic designs by generating systems of mathematical constraints and searching for solutions to mathematical problems with particular design properties and, consequently, aesthetic appeal. Shaffer’s instructional design deliberately incorporated practices of architectural design studios so that student design practices also included public displays (e.g., pinups) and conversations with critics about their evolving designs. This coupling of mathematics and design resulted in increased knowledge about transformational design as well as an appreciation of mathematics as a vehicle for expressive intent.
Studies with younger designers and related electronic technologies also indicate the fruitfulness of design contexts that intersect worlds of artistic expression and mathematical intent. Watt and Shanahan (1994) developed a computer microworld and curriculum materials to support design of quilts via transformational geometry. Research conducted with these tools and materials, together with professional development efforts to help teachers understand children’s thinking, promoted primary grade students’ understanding of transformational geometry, as well as their exploration of algebraic structure, qualities of symmetry, and the limits of induction (Jacobson & Lehrer, 2000; Kaput, 1999; Lehrer, Jacobson, et al., 1998). As with the designers described by Shaffer (1997), children’s conversations often reflected their appreciation of an interaction between mathematics and expressive intent. For example, students debated the qualities of “interesting” design; one student, for example, suggested that some units would be “boring” no matter what transformations or sequences of transformations were applied to make a quilt. He argued that multiple lines of symmetry would restrict the quilt design to simple translation of units (Hartmann & Lehrer, 2000). That is, units with four lines of symmetry restricted the space of possible design. In contrast, asymmetric units allowed for the greatest number of potential designs. Zech et al. (1998) developed dynamic design tools for children’s (Grade 5) expression of architectural designs, such as those of swing sets on playground. Designing blueprints for these architectural challenges served as a forum for exploration of measure, shape, and their relations.
In summary, the development of motion geometry tools and related technologies affords new forms of mathematical expression. The dual expression of the particular and the general, together with experimentation about their relation, creates pedagogical opportunities to orient students toward mathematical argument as explanation, not just verification. Moreover, because these tools create conditions for construction and experimentation about shape and form, students at all ages tend to develop analytic capabilities that have long proven difficult to achieve. Perhaps most exciting is the potential for pedagogy at the boundaries of mathematics and design that capitalizes on the expression of mathematical intent. Of course, mathematical intent, in turn, is supported and shaped by these tools.
Modeling Perspectives
In this section we conclude with an abbreviated tour of some of the emerging work in mathematical modeling in K–12 education. Model-based reasoning is erected on foundations of analogy, representation, and inscription. Analogies, of course, are at the heart of modeling (Hesse, 1965). One system stands in for another. Models are sustained by mappings between the representing and represented worlds, and the nature of these mappings is governed by systems of inscription and notation (Hestenes, 1992). Consider that maps highlight and preserve some aspects of the world while sacrificing others. The familiar Mercator projection facilitates navigation but distorts area of landmasses, often with devastating political consequences.
Bridging Epistemologies
The separation of world and model constitutes a bridge between the epistemologies of mathematics and science. On the one hand, modeling provides opportunities to create coherent and valid mathematical structures. These invite proof so that one can better understand the edifice one is erecting for purposes of representation (Hodgson & Riley, 2001). On the other hand, because models and their referents are distinct, their relation is not one of copy, but rather of fit. Fits between models and worlds are never congruent (the separation between model and world just mentioned), so residuals between them can be determined only in light of other potential models (Grosslight, Unger, & Smith, 1991; Lesh & Doerr, 1998). These qualities of models and modeling practices are at the heart of professional practice in science and in some branches of mathematics (Giere, 1992; Stewart & Golubitsky, 1992).
Research studies of student modeling have generally followed two somewhat overlapping paradigms. The first line of inquiry focuses on model-eliciting problems (Lesh, Hoover, Hole, Kelly, & Post, 2000), in which students invent, revise, and share models as solutions to single problems that typically are solved during one lesson. Problems are often drawn from realms of professional practice, especially engineering, business, and the social sciences. Consequently, they often require students to integrate multiple forms of mathematics, not simply the application of a single solution procedure (see the volume by Doerr & Lesh, in press). The second line of inquiry complements the first by engaging students in the progressive mathematization of nature. For example, students pose questions about motion or biological growth and develop models as explanations (diSessa, 2000; Kaput, Roschelle, & Stroup, 2000; Lehrer & Schauble, 2002). This second strand of research focuses on long-term development of student reasoning because acquiring capabilities and propensities to adopt a modeling stance toward the world is an epistemology with slow evolution. Consequently, research typically spans months or even years of student learning. Both strands of work emphasize the development of modelbased reasoning in contexts designed to support these practices, so they typically require substantial programs of teacher professional development (e.g., Clark & Lesh, 2002; Lehrer & Schauble, 2000; Schorr & Clark, in press). We focus on model-eliciting problems in the remainder of this section to represent this broader spectrum of research.
Cycles of Modeling
At the heart of a modeling perspective is the belief that some of the most important “big ideas” in elementary mathematics are models (or conceptual systems) for making sense of mathematically significant types of situations (Doerr & Lesh, 2002). Model-eliciting problems are designed to evoke mathematical systems, not single procedures, as solutions (Lesh et al., 2000). Students make sense of these situations not all at once, but rather in a cycle of invention and revision (Lesh & Doerr, 1998; Doerr, Post, & Zawojewski, 2002; Lesh & Harel, in press). Figure 15.3 displays one such model-eliciting problem and a case of one group of seventhgrade students’progressive efforts to make sense of this situation. This case illuminates several features of modeling that have been replicated across widely diverse populations of students and problem types (see Doerr & Lesh, 2002, for a compendium of studies).First, student modeling generally occurs through a series of develop-test-revise cycles. Each cycle involves somewhat different ways of thinking about the nature of givens, goals, and possible solution steps (Lesh & Harel, in press). Refinement typically occurs as students attempt to create coherent and consistent mappings between the representing and represented worlds, often by noticing the implications of a particular choice of representation for the world or by noticing how a feature of the world remains unaccounted for in a model system. These observations resulted in several different interpretations across time. Second, recalling our earlier descriptions of inscriptional mediation of mathematical thinking, modeling nearly always involves multiple and interacting systems of inscription and notation as students grapple with potential correspondences between the world and the emerging mathematical description. Third, there nearly always seem to be multiple and often uncoordinated ideas “in the air” during early phases of modeling, and these are reconciled and stabilized as students attempt to fit models to what they consider data. Thus, data and models often codevelop. Fourth, initial efforts to establish fit are nearly always local, and it is the need to consider others’ models and data that often prompts testing for more general structures (Lesh & Doerr, 2002). In the case outlined in Figure 15.3, the group’s model was tested further in light of data gathered and models proposed by other student-modelers. This underscores an expanded sense of mathematical argument as conviction and experiment.
Although this research paper is aimed primarily at student learning, modeling research has prompted the development of new forms of research practices and research design methodologies (Kelly & Lesh, 2000). These arose to enable multiple researchers at distant research sites to coordinate work that employed distinct theoretical perspectives focused on multiple levels of interacting participants (students, teachers, curriculum designers, and researchers; Lesh, in press). Crosssite collaborations were accomplished by using shared tasks and research tools (Lesh, Hoover, Hole, Kelly, & Post, 2001) and by recognizing that all of the relevant participants (researchers, teachers, students, and others) can all be thought of as being in the business of designing models and accompanying conceptual tools to make sense of their experiences. Thus, multiple tiers of analysis were required for the conduct of these studies (Lesh, 2002), a prospect that augments the design study perspective described previously.
Implications
Mathematical thinking is a specialized form of argument and inscription, but it has its genesis in the development of everyday capacities of pretense, possibility, conversation, and inscription. Development of mathematical literacy relies on the design of learning niches that support its continued evolution. Schooling provides an unparalleled opportunity to nurture mathematical thinking because it is one of the few arenas where histories of learning can be systematically supported. Of course, this opportunity is founded on the material support of curriculum, the commitment of teachers as professionals, and the development of knowledge about student thinking and learning in contexts where argument and inscription take center stage. With this in mind, we suggest a few plausible directions for research in mathematics education.
First, we urge consideration of a broader scope of mathematics as worthy of research. Most studies focus on analysis (in later grades) and number concepts (in earlier grades). Although we believe this research has proven productive and valuable, it ignores realms of mathematics that may well prove foundational for a mathematics education. For example, the Elkonin-Davydov approach to elementary mathematics education in Russia takes measurement, not “natural” numbers, as foundational. Hence, in this program children’s early mathematical experiences are oriented toward measure, not count. Other possibilities suggest themselves, such as early and prolonged emphasis on space and geometry and consideration of the roles of modeling and design in the formation of mathematical expression and epistemology.
Second, and following from a broader scope of inquiry, the nature and grounds of professional practice in the community of researchers require fundamental change. Study of the development of mathematical thinking, rather than piecemeal attention to relatively small components, requires considerate crafting of mathematical experience so that learners consistently participate in mathematical argument and expression. In addition, it requires research designs that are coordinated with this craftsmanship to come to understand long-term development. The complexity of this problem suggests a reorganization of professional practices so that design of learning environmentsandstudyofdevelopmentcanbesystematically examined and become coconstituted. This form of research is practiced currently in engineering professions, with their emphasis on design prototypes and iterative design. However, to our knowledge, only embryonic forms of this way of working currently exist in mathematics education research.
Third, and in concert with the previous two suggestions, the focus on a mathematics education needs to be coordinated with other realms of endeavor, recalling that the same child who is learning to participate in mathematical argument is also learning to participate in scientific argument, historical argument, and so on. Each of these forms of literacy has implications for the development of identities and interests, and these should be more systematically scrutinized. Dewey (1938) suggested that identities and interests emerge from personal experience and expression. Hence, if we aim to promote students as authors of mathematical expression, then we need to understand more about how these experiences (which are the objects of instructional design) are coordinated and differentiated during the course of education.
Bibliography:
- Amsel, E., & Smalley, J. D. (2001). Beyond really and truly: Children’s counterfactual thinking about pretend and possible worlds. In P. Mitchell & K. J. Riggs (Eds.), Children’s reasoning and the mind (pp. 121–147). Hove, UK: Taylor and Francis.
- Anderson, J. R., & Schunn, C. D. (2000). Implications of theACT-R learning theory: No magic bullets. In R. Glaser (Ed.), Advances in instructional psychology. Educational design and cognitive science (Vol. 5, pp. 1–33). Mahwah, NJ: Erlbaum.
- Anderson, R. C., Chinn, C., Chang, J., Waggoner, M., & Yi, H. (1997). On the logical integrity of children’s arguments. Cognition and Instruction, 15(2), 135–167.
- Arcavi, A., & Hadas, N. (2000). Computer mediated learning: An example of an approach. International Journal of Computers for Mathematical Learning, 5, 25–45.
- Ball, D. L., & Bass, H. (2000). Making believe: The collective construction of public mathematical knowledge in the elementary classroom. In D. Phillips (Ed.), Yearbook of the national society for the study of education, Constructivism in education (pp. 193–224). Chicago: University of Chicago Press.
- Banchoff, T. F. (1990). Dimension. In L. A. Steen (Ed.), On the shoulders of giants. New approaches to numeracy (pp. 11–59). Washington, DC: National Academy Press.
- Barker, R. G., & Wright, H. F. (1954). Midwest and its children: The psychological ecology of an American town. Evanston, IL: Row, Peterson.
- Baturo, A., & Nason, R. (1996). Student teachers’ subject matter knowledge within the domain of area measurement. Educational Studies in Mathematics, 31, 235–268.
- Benson, D. C. (1999). The moment of proof. Oxford, UK: Oxford University Press.
- Berlinski, D. (2000). The advent of the algorithm. New York: Harcourt.
- Brizuela, B. (1997). Inventions and conventions: A story about capital numbers. For the Learning of Mathematics, 17, 2–6.
- Brown, A. L. (1992). Design experiments: Theoretical and methodological challenges in creating complex interventions in classroom settings. Journal of the Learning Sciences, 2, 141–178.
- Carpenter, T. P., & Lewis, R. (1976). The development of the concept of a standard unit of measure in young children. Journal for Research in Mathematics Education, 7, 53–64.
- Chazan, D. (1993). High school geometry students’ justification for their views of empirical evidence and mathematical proof. Educational Studies in Mathematics, 24, 359–387.
- Chazan, D., & Yerushalmy, M. (1998). Charting a course for secondary geometry. In R. Lehrer & D. Chazan (Eds.), Designing learning environments for developing understanding of geometry and space (pp. 67–90). Mahwah, NJ: Erlbaum.
- Clements, D. H., & Battista, M. A. (1989). Learning of geometric concepts in a LOGO environment. Journal for Research in Mathematics Education, 20, 450–467.
- Clements, D. H., & Battista, M. A. (1990). The effects of LOGO on children’s conceptualizations of angle and polygons. Journal for Research in Mathematics Education, 21, 356–371.
- Clements, D. H., Battista, M. T., & Sarama, J. (1998). Development of geometric and measurement ideas. In R. Lehrer & D. Chazan (Eds.), Designing learning environments for developing understanding of geometry and space (pp. 201–225). Mahwah, NJ: Erlbaum.
- Clements,D.H.,Battista,M.T.,&Sarama,J.(2001). Reston, VA: National Council of Teachers of Mathematics.
- Clements, D. H., Battista, M. T., Sarama, J., Swaminathan, S., & McMillen, S. (1997). Students’ development of length concepts in a Logo-based unit on geometric paths. Journal for Research in Mathematics Education, 28(1), 70–95.
- Clements,D.H.,Sarama,J.,Yelland,N.,&Glass,B.(inpress).Learning and teaching geometry with computers in the elementary and middle school. In K. Heid & G. Blume (Eds.), Research on Technology in the Learning and Teaching of Mathematics: Syntheses and Perspectives. New York: Information Age Publishing, Inc.
- Cobb, P. (2001). Supporting the improvement of learning and teaching in social and institutional context. In S. Carver & D. Klahr (Eds.), Cognition and instruction: Twenty-five years of progress (pp. 455–478). Mahwah, NJ: Erlbaum.
- Cobb, P., Gravemeijer, K., Yackel, E., McClain, K., & Whitenack, J. (1997).Mathematizingandsymbolizing:Theemergenceofchains ofsignificationinonefirst-gradeclassroom.InD.Kirshner&J.A. Whitson (Eds.), Situated cognition: Social, semiotic, and psychological perspectives (pp. 151–233). Mahwah, NJ: Erlbaum.
- Cobb, P., Wood, T., Yackel, E., & McNeal, B. (1992). Characteristics of classroom mathematics traditions: An interactional analysis. American Education Research Journal, 29(3), 573–604.
- Cobb, P., Yackel, E., & Wood, T. (1988). Curriculum and teacher development: Psychological and anthropological perspectives. In E. Fennema, T. P. Carpenter, & S. J. Lamon (Eds.), Integrating research on teaching and learning mathematics (pp. 92–121). Madison: University of Wisconsin.
- Coe, R., & Ruthven, K. (1994). Proof practices and constructs of advanced mathematics students. British Educational Research Journal, 20, 41–53.
- Cohen, S. (1985). The development of constraints on symbolmeaning structure in notation: Evidence from production, interpretation, and forced-choice judgments. Child Development, 56, 177–195.
- Confrey, J. (1995). Student voice in examining “splitting” as an approach to ratio, proportions, and fractions. Paper presented at the 19th International Conference for the Psychology of Mathematics Education, Universidade Federal de Permanbuco, Recife, Brazil.
- Confrey, J., & Smith, E. (1995). Splitting, covariation and their role in the development of exponential functions. Journal for Research in Mathematics Education, 26, 66–86.
- Crosby, A. W. (1997). The measure of reality. Cambridge, UK: Cambridge University Press.
- Darke, I. (1982). A review of research related to the topological primacy hypothesis. Educational Studies in Mathematics, 13, 119–142.
- Davis, P. J., & Hersh, R. (1981). The mathematical experience. Boston: Houghton Mifflin.
- DeLoache, J. S. (1987). Rapid change in symbolic functioning of young children. Science, 238, 1556–1557.
- DeLoache, J. S. (1989). Young children’s understanding of the correspondence between a scale model and a larger space. Cognitive Development, 4, 121–139.
- DeLoache, J. S. (1995). Current understanding and use of symbols: The model model. Current Directions in Psychological Science, 4, 109–113.
- Devaney, R. L. (1998). Chaos in the classroom. In R. Lehrer & D. Chazan (Eds.), Designing learning environments for developing understanding of geometry and space(pp. 91–107). Mahwah, NJ: Erlbaum.
- de Villiers, M. (1998). An alternative approach to proof in dynamic geometry. In R. Lehrer & D. Chazan (Eds.), Designing learning environments for developing understanding of geometry and space (pp. 369–393). Mahwah, NJ: Erlbaum.
- Devlin, K. (2000). The math gene. How mathematical thinking evolved and why numbers are like gossip. New York: Basic Books.
- Dewey, J. (1938). Experience and education. New York: Collier Books.
- Dias, M. G., & Harris, P. L. (1988). The effect of make-believe play on deductive reasoning. British Journal of Developmental Psychology, 6, 207–221.
- Dias, M. G., & Harris, P. L. (1990). The influence of the imagination on reasoning by young children. British Journal of Developmental Psychology, 8, 305–318.
- diSessa, A. (1995). Epistemology and systems design. In A. diSessa, C. Hoyles, R. Noss, & L. D. Edwards (Eds.), Computers and exploratory learning (pp. 15–29). Berlin, Germany: SpringerVerlag.
- diSessa, A. (2000). Changing minds: Computers, learning, and literacy. Carmbridge, MA: MIT Press.
- diSessa, A. A. (in press). Students’ criteria for representational adequacy. In K. Gravemeijer, R. Lehrer, B. van Oers, & L. Verschaffel (Eds.), Symbolizing, modeling and tool use in mathematics education. Dortrecht, The Netherlands: Kluwer.
- diSessa, A., Hammer, D., Sherin, B., & Kolpakowski, T. (1991). Inventing graphing: Metarepresentational expertise in children. Journal of Mathematical Behavior, 10, 117–160.
- Doerr, H., & Lesh, R. (Eds.). (2002). Beyond constructivism: A models and modeling perspective on mathematics teaching, learning, and problem solving. Mahwah, NJ: Erlbaum.
- Drew, P., & Heritage, J. E. (1992). Talk at work. Cambridge, UK: Cambridge University Press.
- Edwards, L. D. (1991). Children’s learning in a computer microworld for transformation geometry. Journal for Research in Mathematics Education, 22, 122–137.
- Edwards, L. D. (1998). Embodying mathematics and science: Microworlds as representations. Journal of Mathematical Behavior, 17, 53–78.
- Edwards, D. (1999). Odds and evens: Mathematical reasoning and informal proof. Journal of Mathematical Behavior, 17, 489–504.
- Ellis, S., Siegler, R. S., & Van Voorhis, F. E. (2000). Developmental changes in children’s understanding of measurement procedures and principles. Unpublished manuscript.
- Forman, E. A. (in press). A sociocultural approach to mathematics reform: Speaking, inscribing, and doing mathematics within communities of practice. In J. Kilpatrick, G. Martin, & D. Shifter (Eds.), A research companion to the Standards and Principles. Reston, VA: National Council of Teachers of Mathematics.
- Forman, E. A., Larreamendy-Joerns, J., Stein, M. K., & Brown, C. A. (1998). “You’re going to want to find out which and prove it”: Collective argumentation in a mathematics classroom. Learning and Instruction, 8, 527–548.
- Forrester, M. A., & Pike, C. D. (1998). Learning to estimate in the mathematics classroom: A conversation-analytic approach. Journal for Research in Mathematics Education, 29, 334–356.
- Gee, J. P. (1997). Thinking, learning, and reading: The situated sociocultural mind. In D. Kirshner & J. A. Whitson (Eds.), Situated cognition: Social, semiotic, and psychological perspectives (pp. 235–259). Mahwah, NJ: Erlbaum.
- Gee, J. P. (in press). Critical discourse analysis. In R. Beach, J. Green, M. Kamil, & T. Shanahan (Eds.), Multidisciplinary perspectives on literacy research. Cresskill, NJ: Hampton Press.
- Gentner, D. (1983). Structure-mapping: Atheoretical framework for analogy. Cognitive Science, 7, 155–170.
- Gentner, D., & Loewenstein, J. (2002). Relational language and relational thought. In E. Amsel & J. P. Byrnes (Eds.), Language, literacy, and cognitive development. The development and consequences of symbolic communication (pp. 87–120). Mahwah, NJ: Erlbaum.
- Gentner, D., & Toupin, C. (1986). Systematicity and surface similarity in the development of analogy. Cognitive Science, 10, 277–300.
- Giere, R. N. (1992). Cognitive models of science. Minneapolis: University of Minnesota Press.
- Goldenberg, E. P., Cuoco, A. A., & Mark, J. (1998). Arole for geometry in general education. In R. Lehrer & D. Chazan (Eds.), Designing learning environments for developing understanding of geometry and space (pp. 3–44). Mahwah, NJ: Erlbaum.
- Goodman, N. (1976). Languages of art. Indianapolis, IN: Hackett.
- Goodnow, J. (1977). Children’s drawings. Cambridge, MA: Harvard University Press.
- Goodwin, C. (1994). Professional vision. American Anthropologist, 96(3), 606–633.
- Goodwin, C. (2000). Practices of color classification. Mind, culture, and activity, 7(1 & 2), 19–36.
- Greeno, J. G. (1998). The situativity of knowing, learning, and research. American Psychologist, 53, 5–26.
- Hall, R. (1990). Making mathematics on paper: Constructing representations of stories about related linear functions. Unpublished doctoral dissertation, University of California at Irvine.
- Hall, R. (1996). Representation as shared activity: Situated cognition and Dewey’s cartography of experience. The Journal of the Learning Sciences, 5(3), 209–238.
- Hall, R. (1999). The organization and development of discursive practices for “having a theory.” Discourse Processes, 27, 187– 218.
- Hall, R., Stevens, R., & Torralba, T. (in press). Disrupting representational infrastructure in conversations across disciplines. Mind, Culture, and Activity, 9(3).
- Halliday, M. A. K. (1978). Sociolinguistics aspects of mathematical education.InM.Halliday(Ed.),Languageassocialsemiotic:The social interpretation of language and meaning (pp. 195–204). London: University Park Press.
- Hanna, G. (1991). Mathematical proof. In D. Tall (Ed.), Advanced mathematical thinking (pp. 54–61). Dordrecht, The Netherlands: Kluwer Academic Publishers.
- Hanna, G. (1995). Challenges to the importance of proof. For the Learning of Mathematics, 15, 42–49.
- Harel, G. (1998). Greek versus modern mathematical thought and the role of Aristotelian causality in the mathematics of the Renaissance: Sources for understanding epistemological obstacles in college students’conceptions of proof. Plenary talk given at the International LinearAlgebra Society Conference, Madison, WI.
- Harel, G., & Sowder, L. (1998). Students’ proof schemes. In E. Dubinsky, A. Schoenfeld, & J. Kaput (Eds.), Research on collegiate mathematics education (Vol. 3, pp. 234–283). American Mathematical Society.
- Harel,I.,&Papert,S.(1991).Norwood,NJ:Ablex.
- Harris, P. J., & Leevers, H. J. (2001). Reasoning from false premises. In P. Mitchell & K. J. Riggs (Eds.), Children’s reasoning and the mind (pp. 67–86). UK: Psychology Press, Taylor and Francis.
- Hartmann, C., & Lehrer, R. (2000). Quilt design as incubator for geometric ideas and mathematical habits of mind. Proceedings of the 22nd annual meeting of the North American Chapter of the International Group for the Psychology of Mathematics Education, Tucson, AZ.
- Hatano, G., & Ito, Y. (1965). Development of length measuring behavior. Japanese Journal of Psychology, 36, 184–196.
- Haverty, L. A., Koedinger, K. R., Klahr, D., & Alibali, M. W. (2000). Solving inductive reasoning problems in mathematics: Not-so-trivial pursuit. Cognitive Science, 24, 249–298.
- Hawkins, J., Pea, R. D., Glick, J., & Scribner, S. (1984). “Merds that laugh don’t like mushrooms.” Developmental Psychology, 20, 584–594.
- Healy, L., & Hoyles, C. (2000). A study of proof conceptions in algebra. Journal for Research in Mathematics Education, 31, 396–428.
- Henningsen, M., & Stein, M. K. (1997). Mathematical tasks and student cognition: Classroom-based factors that support and inhibit high-level mathematical thinking and reasoning. Journal for Research in Mathematics Education, 28(5), 524–549.
- Herbst, P. (2002). Understanding the work of the teacher getting students to prove. Journal of Research in Mathematics Education, 33, 176–203.
- Hersh, R. (1993). Proving is convincing and explaining. Educational Studies in Mathematics, 24(4), 389–399.
- Hershkowitz, R., & Schwarz, B. B. (1999). Reflective processes in a mathematics classroom with a rich learning environment. Cognition and Instruction, 17, 65–91.
- Hesse, M. B. (1965). Forces and fields. Totowa, NJ: Littlefield, Adams.
- Hestenes, D. (1992). Modeling games in the Newtonian world. American Journal of Physics, 60, 440–454.
- Hiebert, J. (1981a). Cognitive development and learning linear measurement. Journal for Research in Mathematics Education, 12, 197–211.
- Hiebert, J. (1981b). Units of measure: Results and implications from national assessment. Arithmetic Teacher, 28, 38–43.
- Hiebert, J. (1984). Why do some children have trouble learning measurement concepts? Arithmetic Teacher, 31, 19–24.
- Hildreth, D. J. (1983). The use of strategies in estimating measurements. Arithmetic Teacher, 30, 50–54.
- Hodgson, T., & Riley, K. J. (2001). Real-world problems as contexts for proof. Mathematics Teacher, 94(9), 724–728.
- Hoyles, C. (1997). The curricular shaping of students’approaches to proof. For the Learning of Mathematics, 17, 7–16.
- Izsak, A. (2000). Inscribing the winch: Mechanisms by which students develop knowledge structures for representing the physical world with algebra. The Journal of the Learning Sciences, 9(1), 31–74.
- Jackiw, N. (1995). The geometer’s sketchpad. Berkeley, CA: Key Curriculum Press.
- Jacobson, C., & Lehrer, R. (2000). Teacher appropriation and student learning of geometry through design. Journal for Research in Mathematics Education, 31, 71–88.
- Joram, E., Subrahmanyam, K., & Gelman, R. (1998). Measurement estimation: Learning to map the route from number to quantity and back. Review of Educational Research, 68, 413–449.
- Jorgensen,J.C.,&Falmagne,R.J.(1992).Aspectsofthemeaningof if…thenforolderpreschoolers:Hypotheticality,entailment,and suppositional processes. Cognitive Development, 7, 189–212.
- Kaiser, D. (2000). Stick-figure realism: Conventions, reification, and the persistence of Feynman diagrams, 1948–1964. Representations, 70, 49–86.
- Kaput, J. (1992). Technology and mathematics education. In D. A. Grouws (Ed.), Research on mathematics teaching and learning (pp. 515–556). New York: Macmillan.
- Kaput, J. (1999). Teaching and learning a new algebra. In E. Fennema & T. A. Romberg (Eds.), Mathematics classrooms that promote understanding (pp. 133–155). Mahwah, NJ: Erlbaum.
- Kaput, J., & Shaffer, D. (in press). On the development of human representational competence from an evolutionary point of view. In K. Gravemeijer, R. Lehrer, B. Van Oers, & L. Verschaffel (Eds.), Symbolizing, modeling, and tool use in mathematics education (pp. 269–286). Dordrecht, The Netherlands: Kluwer Academic.
- Karmiloff-Smith, A. (1992). Beyond modularity. Cambridge, MA: MIT Press.
- Kelly, A. E., & Lesh, R. A. (Eds.). (2000). Handbook of research design in mathematics and science education. Mahwah, NJ: Erlbaum.
- Kemeny, V. (2001). Discursive construction of mathematical meaning: A study of teaching mathematics through conversation in the primary grades. Unpublished doctoral dissertation, University of Wisconsin, Madison.
- Kerr, D. R., & Lester, S. K. (1976). An error analysis model for measurement. In D. Nelson & R. E. Reys (Eds.), Measurement in school mathematics (pp. 105–122). Reston, VA: National Council of Teachers of Mathematics.
- Kline, M. (1980). Mathematics: The loss of certainty. Oxford, UK: Oxford University Press.
- Koedinger, K. R. (1998). Conjecturing and argumentation in highschool geometry students. In R. Lehrer & D. Chazan (Eds.), Designing learning environments for developing understanding of geometry and space (pp. 319–347). Mahwah, NJ: Erlbaum.
- Koedinger, K. R., & Anderson, J. R. (1990). Abstract planning and perceptual chunks: Elements of expertise in geometry. Cognitive Science, 14, 511–550.
- Konold, C., & Pollatsek, A. (in press). Data analysis as the search for signals in noisy processes. Journal for Research in Mathematics Education.
- Kotovsky, L., & Gentner, D. (1996). Comparison and categorization in the development of relational similarity. Child Development, 67, 2797–2822.
- Krummerheuer, G. (1995). The ethnography of argumentation. In P. Cobb & H. Bauersfeld (Eds.), The emergence of mathematical meaning (pp. 229–269). Mahwah, NJ: Erlbaum.
- Krummerheuer, G. (1998). Formats of argumentation in the mathematics classroom. In H. Steinbring, M. G. Bartolini Bussi, & A. Sierpinska (Eds.), Language and communication in the mathematics classroom (pp. 223–234). Reston, VA: National Council of Teachers of Mathematics.
- Kuhn, D. (1977). Conditional reasoning in children. Developmental Psychology, 13, 342–353.
- Kuhn, D. (1989). Children and adults as intuitive scientists. Psychological Review, 96, 674–689.
- Kuhn, D. (1991). The skills of argument. Cambridge, UK: Cambridge University Press.
- Kuhn, D. (1992). Thinking as argument. Harvard Educational Review, 62, 155–178.
- Kuhn, D. (2001). How do people know? Psychological Science, 12(1), 1–8.
- Kuhn, D., Amsel, E., & O’Loughlin, M. (1988). The development of scientific thinking skills. New York: Academic Press.
- Kuhn, D., Shaw, V., & Felton, M. (1997). Effects of dyadic instruction on argumentative reasoning. Cognition and Instruction, 15, 287–315.
- Lakatos, I. (1976). Proofs and refutations. Cambridge, UK: Cambridge University Press.
- Lakoff, G., & Nunez, R. E. (1997). The metaphorical structure of mathematics: Sketching out cognitive foundations for a mindbased mathematics. In L. D. English (Ed.), Mathematical reasoning.Analogies,metaphors,andimages(pp.21–89).Mahwah,NJ: Erlbaum.
- Lakoff, G., & Nunez, R. E. (2000). Where mathematics comes from. New York: Basic Books.
- Lampert, M. (2001). Teaching problems and the problems of teaching. New Haven, CT: Yale University Press.
- Lampert, M., Rittenhouse, P., & Crumbaugh, C. (1996). Agreeing to disagree: Developing sociable mathematical discourse. In D. Olson & N. Torrance (Eds.), The handbook of education and human development (pp. 731–764). Cambridge, MA: Blackwell.
- Latour, B. (1986). Visualization and cognition: Thinking with eyes and hands. Knowledge and Society: Studies in the Sociology of Culture Past and Present, 6, 1–40.
- Latour, B. (1987). Science in action: How to follow scientists and engineers through society. Cambridge, MA: Harvard University Press.
- Lee, K., Karmiloff-Smith, A., Cameron, C. A., & Dodsworth, P. (1998). Notational adaptation in children. Canadian Journal of Behavioural Science, 30, 159–171.
- Lehrer, R. (2002). Developing understanding of measurement. In J. Kilpatrick, G. Martin, & D. Schifter (Eds.), A research companion to the Standards and Principles. Reston, VA: National Council of Teachers of Mathematics.
- Lehrer, R., Guckenberg, T., & Lee, O. (1988a). Comparative study of the cognitive consequences of inquiry-based Logo instruction. Journal of Educational Psychology, 80(4), 543–553.
- Lehrer, R., Jacobson, C., Kemeny, V., & Strom, D. (1999). Building on children’s intuitions to develop mathematical understanding ofspace. InE. Fennema &T.A. Romberg (Eds.), Classroomsthat promote mathematical understanding (pp. 63–87). Mahwah, NJ: Erlbaum.
- Lehrer,R.,Jacobson,C.,Thoyre,G.,Kemeny,V.,Strom,D.,Horvath, J.,Gance,S.,&Koehler,M.(1998).Developingunderstandingof geometry and space in the primary grades. In R. Lehrer & D. Chazan (Eds.), Designing learning environment for developing understanding of geometry and space (pp. 169–200). Mahwah, NJ: Erlbaum.
- Lehrer, R., Jenkins, M., & Osana, H. (1998). Longitudinal study of children’s reasoning about space and geometry. In R. Lehrer & D. Chazan (Eds.), Designing learning environment for developing understanding of geometry and space (pp. 137–167). Mahwah, NJ: Erlbaum.
- Lehrer, R., & Pritchard, C. (in press). Symbolizing space into being. In K. Gravemeijer, R. Lehrer, B. Van Oers, & L. Verschaffel (Eds.), Symbolizing, modeling, and tool use in mathematics education. Dordrecht, The Netherlands: KluwerAcademic Press.
- Lehrer, R., Randle, L., & Sancilio, L. (1989). Learning pre-proof geometry with LOGO. Cognition and Instruction, 6, 159–184.
- Lehrer, R., & Schauble, L. (2000). Modeling in mathematics and science. In R. Glaser (Ed.), Advances in Instructional Psychology (pp. 101–159). Mahwah, NJ: Erlbaum.
- Lehrer, R., & Schauble, L. (2002). Symbolic communication in mathematics and science: Co-constituting inscription and thought. In E. Amsel & J. Byrnes (Eds.), The development of symbolic communication (pp. 167–192). Mahwah, NJ: Erlbaum.
- Lehrer, R., Schauble, L., Carpenter, S., & Penner, D. E. (2000). The inter-related development of inscriptions and conceptual understanding. In P. Cobb, E. Yackel, & K. McClain (Eds.), Symbolizing and communicating in mathematics classrooms: Perspectives on discourse, tools, and instructional design (pp. 325–360). Mahwah, NJ: Erlbaum.
- Lehrer, R., Strom, D., & Confrey, J. (in press). Grounding metaphors and inscriptional resonance: Children’s emerging understanding of mathematical similarity. Cognition and Instruction.
- Leinhardt, G., & Schwarz, B. B. (1997). Seeing the problem: An explanation from Polya. Cognition and Instruction, 15, 395–434.
- Lesh, R. (2002). Research design in mathematics education: Focusing on design experiments. In L. English (Ed.), The international handbook of research design in mathematics education (pp. 241– 287). Hillsdale, N.J.: Erlbaum.
- Lesh, R., & Doerr, H. (1998). Symbolizing, communicating, and mathematizing: Key components of models and modeling. In P. Cobb & E. Yackel (Eds.), Symbolizing and communicating in mathematics classrooms (pp. 361–383). Mahwah, NJ: Erlbaum.
- Lesh, R., & Harel, G. (in press). Problem solving, modeling and local conceptual development. Models and modeling in mathematics education [Monograph for International Journal for Mathematical Thinking and Learning]. Hillsdale, NJ: Erlbaum.
- Lesh, R., Hoover, M., Hole, B., Kelly, A., & Post, T. (2000). Principles for developing thought revealing activities for students and teachers. In A. Kelly & R. Lesh (Eds.), The handbook of research design in mathematics and science education (pp. 591–646). Hillsdale, NJ: Erlbaum.
- Leslie, A. M. (1987). Pretense and representation: The origins of “theory of mind.” Psychological Review, 94, 412–426.
- Levi, I. (1996). For the sake of the argument. Cambridge, England: Cambridge University Press.
- Lindquist, M. (1989). The measurement standards. Arithmetic Teacher, 37, 22–26.
- Lynch, M. (1990).The externalized retina: Selection and mathematization in the visual documentation of objects in the life sciences. In M. Lynch & S. Woolgar (Eds.), Representation in scientific practice (pp. 153–186). Cambridge, MA: MIT Press.
- Martin, W. G., & Harel, G. (1989). Proof frames of preservice elementary teachers. Journal for Research in Mathematics Education, 20, 41–51.
- McClain, K., & Cobb, P. (2001). An analysis of development of sociomathematical norms in one first-grade classroom. Journal for Research in Mathematics Education, 32, 236–266.
- McClain, K., Cobb, P., Gravemeijer, K., & Estes, B. (1999). Developing mathematical reasoning within the context of measurement. In L. V. Stiff & F. R. Curcio (Eds.), Developing mathematical reasoning in grades K-12 (pp. 93–106). Reston, VA: National Council of Teachers of Mathematics.
- Meira, L. (1995). The microevolution of mathematical representations in children’s activity. Cognition and Instruction, 13, 269– 313.
- Meira, L. (in press). Mathematical representations as systems of notations-in-use. In K. Gravemeijer, R. Lehrer, B. Van Oers, & L. Verschaffel (Eds.),Symbolizing, modeling, and tool use in mathematics education (pp. 89–106). Dordrecht, The Netherlands: Kluwer.
- Miller, C. S., Lehman, J. F., & Koedinger, K. R. (1999). Goals and learning in microworlds. Cognitive Science, 23, 305–336.
- Miller, K. F. (1984). Child as the measurer of all things: Measurement procedures and the development of quantitative concepts. In C. Sophian (Ed.), Origins of cognitive skills (pp. 193–228). Hillsdale, NJ: Erlbaum.
- Miller, K. F., & Baillargeon, R. (1990). Length and distance: Do preschoolers think that occlusion bring things together? Developmental Psychology, 26, 103–114.
- Moschkovich, J. N. (1996). Moving up and getting steeper: Negotiating shared descriptions of linear graphs. The Journal of the Learning Sciences, 5, 239–277.
- Moss, J., & Case, R. (1999). Developing children’s understanding of the rational numbers: A new model and an experimental curriculum. Journal for Research in Mathematics Education, 30, 122–147.
- Munn, P. (1998). Symbolic function in pre-schoolers. In C. Donlan (Ed.), The development of mathematical skills (pp. 47–71). Hove, UK: Psychology Press, Taylor & Francis.
- Nemirovsky, R., & Monk, S. (2000). “If you look at it the other way . . .”: An exploration into the nature of symbolizing. In P. Cobb, E. Yackel, & K. McClain (Eds.), Symbolizing and communicating in mathematics classrooms. Perspectives on discourse, tools, and instructional design (pp. 177–221). Mahwah, NJ: Erlbaum.
- Nemirovsky, R., Tierney, C., & Wright, T. (1998). Body motion and graphing. Cognition and Instruction, 16, 119–172.
- Newcombe, N. S., & Huttenlocher, J. (2000). Making space. Cambridge, MA: MIT Press.
- Noss, R., & Hoyles, C. (1996). Windows on mathematical meaning. Amsterdam: Kluwer Academic.
- Nunes, T. (1999). Mathematics learning as the socialization of the mind. Mind, Culture, and Activity, 6, 33–52.
- Nunes, T., Light, P., & Mason, J. (1993). Tools for thought: The measurement of length and area. Learning and Instruction, 3, 39–54.
- O’Brien, D., Dias, M., Roazzi, A., & Braine, M. (1998). Conditional reasoning: The logic of supposition and children’s understanding of pretense. In M. D. S. Braine & D. P. O’Brien (Eds.), Mental logic (pp. 245–272). Mahwah, NJ: Erlbaum.
- Ochs, E., Jacoby, S., & Gonzales, P. (1994). Interpretive journeys: How physicists talk and travel through graphic space. Configurations, 2, 151–171.
- Ochs, E., Taylor, C., Rudolph, D., & Smith, R. (1992). Storytelling as a theory-building activity. Discourse Processes, 15, 37–72.
- O’Connor,M.C.,&Michaels,S.(1993).Aligningacademictaskand participation status through revoicing: Analysis of a classroom discourse. Anthropology and Education Quarterly, 24, 318–335.
- O’Connor, M. C., & Michaels, S. (1996). Shifting participant frameworks: Orchestrating thinking practices in group discussion. In D. Hicks (Ed.), Discourse, learning, and schooling (pp. 63–103). Cambridge, UK: Cambridge University Press.
- Olson,D.R.(1994).Cambridge,UK:Cambridge University Press.
- Papert, S. (1980). Mindstorms: Children, computers, and powerful ideas. New York: Basic Books.
- Papert, S., Watt, D., diSessa, A., & Weir, S. (1979). Final report of the Brookline Logo Project: Pt. II. Project summary and data analysis (Logo Memo No. 53). Cambridge, MA: MIT, Artificial Intelligence Laboratory.
- Penner, E., & Lehrer, R. (2000). The shape of fairness. Teaching Children Mathematics, 7, 210–214.
- Petrosino, A., Lehrer, R., & Schauble, L. (in press). Structuring error and experimental variation as distribution in the fourth grade.
- Mathematical Thinking and Learning.
- Piaget, J., Inhelder, B., & Szeminska, A. (1960). The child’s conception of geometry. New York: Harper and Row.
- Pimm, D. (1987). Speaking mathematically: Communication in mathematics classrooms. London: Routledge & Kegan Paul.
- Polya,G.(1945).Princeton,NJ:PrincetonUniversity Press.
- Porter, T. M. (1986). The rise of statistical thinking 1820–1900. Princeton, NJ: Princeton University Press.
- Pozzi, S., Noss, R., & Hoyles, C. (1998). Tools in practice, mathematics in use. Educational Studies in Mathematics, 36, 105–122.
- Resnick, M. (1994). Turtles, termites, and traffic jams. Cambridge, MA: MIT Press.
- Rips, L. J. (1998). Reasoning and conversation. Psychological Review, 105, 411–441.
- Rips, L. J., Brem, S. K., & Bailenson, J. N. (1999). Reasoning dialogues. Current Directions in Psychological Science, 8, 172– 177.
- Roese, N. (1997). Counterfactual thinking. Psychological Bulletin, 121, 133–148.
- Roth, W. M., & McGinn, M. K. (1998). Inscriptions: Toward a theory of representing as social practice. Review of Educational Research, 68, 35–59.
- Rotman, B. (1988). Toward a theory of semiotics of mathematics. Semiotica, 72, 1–35.
- Rotman, B. (1993). Ad Infinitum. Stanford, CA: Stanford University Press.
- Schauble, L. (1996). The development of scientific reasoning in knowledge-rich contexts. Developmental Psychology, 32, 102–119.
- Schoenfeld, A. H. (1988). When good teaching leads to bad results: The disasters of “well taught” mathematics courses. Educational Psychologist, 23, 145–166.
- Schoenfeld, A. H. (1992). Learning to think mathematically: Problem solving, metacognition, and sense making in mathematics. In D. A. Grouws (Ed.), Handbook of research on mathematics teaching and learning (pp. 334–370). New York: Macmillan.
- Schoenfeld, A. H. (1994). What do we know about mathematics curricula? Journal of Mathematical Behavior, 13, 55–80.
- Schoenfeld, A. H., Smith, J. P., III, & Arcavi, A. (1993). Learning: The microgenetic analysis of one student’s evolving understanding of a complex subject matter domain. In R. Glaser (Ed.), Advances in instructional psychology (pp. 55–175). Hillsdale, NJ: Erlbaum.
- Schorr, R., & Clark, K. (in press). Using a modeling approach to analyze the ways in which teachers consider new ways to teach mathematics: Models and modeling in mathematics education [Monograph for International Journal for Mathematical Thinking and Learning]. Hillsdale, NJ: Erlbaum.
- Schunn, C. D., & Anderson, J. R. (1999). The generality/specificity of expertise in scientific reasoning. Cognitive Science, 23, 337–370.
- Scott, F. J., Baron-Cohen, S., & Leslie, A. (1999). “If pigs could fly”: A test of counterfactual reasoning and pretense in children with autism. British Journal of Developmental Psychology, 17, 349–362.
- Segal, J. (2000). Learning about mathematical proof: Conviction and validity. Journal of Mathematical Behavior, 18, 191– 210.
- Senechal, M. (1990). Shape. In L. A. Steen (Ed.), On the shoulders of giants. New approaches to numeracy (pp. 139–181). Washington, DC: National Academy Press.
- Sfard, A. (2000). Symbolizing mathematical reality into being—Or how mathematical discourse and mathematical objects create each other. In P. Cobb, E. Yackel, & K. McClain (Eds.), Symbolizing and communicating in mathematics classrooms. Perspectives on discourse, tools, and instructional design (pp. 37–98). Mahwah, NJ: Erlbaum.
- Sfard, A., & Kieran, C. (2001). Cognition as communication: Rethinking learning-by-talking through multi-faceted analysis of students’mathematical interactions. Mind, Culture, and Activity, 8, 42–76.
- Shaffer, D. W. (1997). Learning mathematics through design: The anatomy of Escher’s world. Journal of Mathematical Behavior, 16, 95–112.
- Shaffer, D. W. (1998). Expressive mathematics: Learning by design. Unpublished doctoral dissertation, MIT, Cambridge, MA.
- Sherin, B. L. (2001). A comparison of programming languages and algebraic notation as expressive languages for physics. International Journal of Computers for Mathematical Learning, 6, 1–61.
- Simon, M. A., & Blume, G. W. (1996). Justification in the mathematics classroom: A study of prospective elementary teachers. Journal for Research in Mathematics Education, 15, 3–31.
- Stevens, R., & Hall, R. (1998). Disciplined perception: Learning to see in technoscience. In M. Lampert & M. L. Blunk (Eds.), Talking mathematics (pp. 107–149). Cambridge, UK: Cambridge University Press.
- Stewart, I. (1975). Concepts of modern mathematics. New York: Dover.
- Stewart, I. (1998). Life’s other secret. New York: Wiley.
- Stewart, I., & Golubitsky, M. (1992). Fearful symmetry: Is God a geometer? London: Penguin Books.
- Strom, D., Kemeny, V., Lehrer, R., & Forman, E. (2001). Visualizing the emergent structure of children’s mathematical argument. Cognitive Science, 25, 733–773.
- Strom, D., & Lehrer, R. (1999). The epistemology of generalization. Paper presented at the Annual Meeting of the American Educational Research Association, Montreal, Quebec, Canada.
- Taplin, J. E., Staudenmayer, H., & Taddonio, J. L. (1974). Developmental changes in conditional reasoning: Linguistic or logical? Experimental Child Psychology, 17, 360–373.
- Thompson, P. W. (1992). Notations, conventions, and constraints: Contributions to effective use of concrete materials in elementary mathematics education. Journal for Research in Mathematics Education, 23(2), 123–147.
- Thurston, W. P. (1995). On proof and progress in mathematics. For the Learning of Mathematics, 15(1), 29–37.
- Toulmin, S. E. (1958). The uses of argument. Cambridge, England: Cambridge University Press.
- van Eemeren, F. H., Grootendorst, R., Henekemans, F. S., Blair, A., Johnson, R. H., Krabbe, E. C., Walton, D. N., Willard, C. A., Woods, J., & Zarefsky, D. (1996). Fundamentals of argumentation theory. Mahwah, NJ: Erlbaum.
- van Oers, B. (2000). The appropriation of mathematical symbols: A psychosemiotic approach to mathematics learning. In E. Y. P. Cobb & K. McClain (Ed.), Symbolizing and communicating in mathematics classrooms: Perspectives on discourse, tools, and instructional design (pp. 133–176). Mahwah, NJ: Erlbaum.
- vanOers,B.(inpress).Themathematizationofyoungchildren’slanguage.InK.Gravemeijer,R.Lehrer,B.vanOers,&L.Verschaffel (Eds.), Symbolizing, modeling, and tool use in mathematics education. Dortrecht,The Netherlands: KluwerAcademic.
- Varelas, M. (1997). Third and fourth graders’ conceptions of repeated trials and best representatives in science experiments. Journal of Research in Science Teaching, 34, 853–872.
- Vygotsky, L. (1978). Mind in society. The development of higher psychological processes. Cambridge, MA: Harvard University Press.
- Warren, B., Ballenger, C., Ogonowski, M., Rosebery, A. S., & Hudicourt-Barnes, J. (2001). Rethinking diversity in learning science: The logic of everyday sense-making. Journal of Research in Science Teaching, 38, 529–552.
- Watt, D. L. (1998). Mapping the classroom using a CAD program: Geometry as applied mathematics. In R. Lehrer & D. Chazan (Eds.), Designing learning environments for developing understanding of geometry and space (pp. 419–438). Mahwah, NJ: Erlbaum.
- Watt, D., & Shanahan, S. (1994). Math and More 2—Teacher Guide. Atlanta, GA: International Business Machines.
- Wells, G. (1999). Dialogic inquiry. Cambridge, UK: Cambridge University Press.
- Wertsch, J. V. (1998). Mind as action. New York: Oxford University Press.
- Wilensky, U., & Resnick, M. (1999). Thinking in levels: A dynamic systems approach to making sense of the world. Journal of Science Education and Technology, 8, 3–18.
- Wood, T. (1999). Creating a context for argument in mathematics class. Journal for Research in Mathematics Education, 30(2), 171–191.
- Yackel, E., & Cobb, P. (1996). Sociomath norms, argumentation, and autonomy in mathematics. Journal for Research in Mathematics Education, 27, 458–477.




